Advertisements
Advertisements
प्रश्न
In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

उत्तर
Area of the shaded region can be calculated as shown below,
Area of the shaded region = Area of equilateral triangle − 3` xx`area of circular arc
`"∴ Area of the shaded region"=sqrt3/4xx8xx8-3xx60/360xxpixx4xx4`
`"∴ Area of the shaded region"=sqrt3xx2xx8-3xx1/6xxpixx4xx4`
`"∴ Area of the shaded region"=sqrt3xx16-1/2xxpixx16`
`"∴ Area of the shaded region"=sqrt3xx16-pixx8`
Substituting sqrt`3=1.732` and `pi=3.142`we get,
`"∴ Area of the shaded region"=1732xx16-3.142xx8`
`"∴ Area of the shaded region"=27.712-25.136`
`"∴ Area of the shaded region"=2.576`
Therefore, area of the shaded region is `2.576 cm^2`
APPEARS IN
संबंधित प्रश्न
The circumference of a circle is 31.4 cm. Find the radius and the area of the circle? (Take π = 3.14)
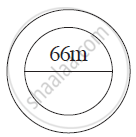
A circular flower bed is surrounded by a path 4 m wide. The diameter of the flower bed is 66 m. What is the area of this path? (π = 3.14)
Find the length of the hypotenuse of an isosceles right-angled triangle whose area is `200^2` cm . Also, find its perimeter
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the sector formed by the arc. (Use π = `22/7`)
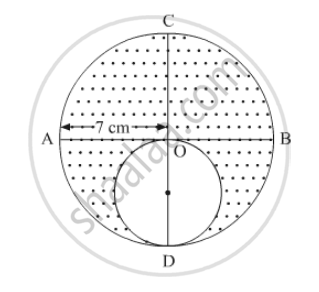
In the following figure, AB and CD are two diameters of a circle perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.
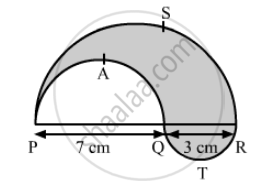
In the the following figure, PSR, RTQ and PAQ are three semicircles of diameter 10 cm, 3 cm and 7 cm respectively. Find the perimeter of shaded region.
If the area of a sector of a circle is `5/18` of the area of the circle, then the sector angle is equal to
Find the area of the shaded region in the given figure, where a circular arc of radius 6 cm has been drawn with vertex of an equilateral triangle of side 12 cm as centre and a sector of circle of radius 6 cm with centre B is made.
The area of the sector of angle θ° of a circle with radius R is
How much distance, in metres, a wheel of 25 cm radius will cover if it rotates 350 times?
