Advertisements
Advertisements
प्रश्न
In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

उत्तर
Area of the shaded region can be calculated as shown below,
Area of the shaded region = Area of equilateral triangle − 3` xx`area of circular arc
`"∴ Area of the shaded region"=sqrt3/4xx8xx8-3xx60/360xxpixx4xx4`
`"∴ Area of the shaded region"=sqrt3xx2xx8-3xx1/6xxpixx4xx4`
`"∴ Area of the shaded region"=sqrt3xx16-1/2xxpixx16`
`"∴ Area of the shaded region"=sqrt3xx16-pixx8`
Substituting sqrt`3=1.732` and `pi=3.142`we get,
`"∴ Area of the shaded region"=1732xx16-3.142xx8`
`"∴ Area of the shaded region"=27.712-25.136`
`"∴ Area of the shaded region"=2.576`
Therefore, area of the shaded region is `2.576 cm^2`
APPEARS IN
संबंधित प्रश्न
A circle of radius 2 cm is cut out from a square piece of an aluminium sheet of side 6 cm. What is the area of the left over aluminium sheet? (Take π = 3.14)
A room 4.9 m long and 3.5 m board is covered with carpet, leaving an uncovered margin of 25 cm all around the room. If the breadth of the carpet is 80 cm, find its cost at ₹ 80 per metre.
The dimensions of a room are 14 m x 10 m x 6.5 m There are two doors and 4 windows in the room. Each door measures 2.5 m x 1.2 m and each window measures 1.5 m x 1 m. Find the cost of painting the four walls of the room at ₹ `35perm^2` .
Find the area of the largest triangle that can be inscribed in a semi-circle of radius runits.
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
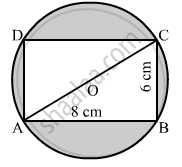
Find the area of the shaded region in the given figure, if ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle.
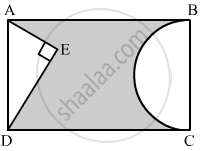
In the given figure, ABCD is a rectangle with AB = 80 cm and BC = 70 cm, ∠AED = 90° and DE = 42 cm. A semicircle is drawn, taking BC as diameter. Find the area of the shaded region.
A square is inscribed in a circle of radius 7 cm. Find the area of the square.
The diameters of three circles are in the ratio 3: 5: 6. If the sum of the circumferences of these circles is 308 cm; find the difference between the areas of the largest and the smallest of these circles.
The formula used to find the area of the circle is ________ sq.units
