Advertisements
Online Mock Tests
Chapters
2: Polynomials
3: Pair of Linear Equations in Two Variables
4: Quadratic Equations
5: Arithmetic Progression
6: Co-Ordinate Geometry
7: Triangles
8: Circles
9: Constructions
10: Trigonometric Ratios
11: Trigonometric Identities
12: Trigonometry
▶ 13: Areas Related to Circles
14: Surface Areas and Volumes
15: Statistics
16: Probability
![RD Sharma solutions for Mathematics [English] Class 10 chapter 13 - Areas Related to Circles RD Sharma solutions for Mathematics [English] Class 10 chapter 13 - Areas Related to Circles - Shaalaa.com](/images/8193647920-mathematics-english-class-10_6:5809898a5fef45e9a2f7e6b414d392fa.jpg)
Advertisements
Solutions for Chapter 13: Areas Related to Circles
Below listed, you can find solutions for Chapter 13 of CBSE RD Sharma for Mathematics [English] Class 10.
RD Sharma solutions for Mathematics [English] Class 10 13 Areas Related to Circles Exercise 13.1 [Pages 12 - 13]
Find the circumference and area of circle of radius 4.2 cm
Find the circumference of a circle whose area is 301.84 cm2.
Find the area of circle whose circumference is 44 cm.
The circumference of a circle exceeds diameter by 16.8 cm. Find the circumference of
circle.
A horse is tied to a pole with 28m long string. Find the area where the horse can graze.
A steel wire when bent is the form of square encloses an area of 12 cm2. If the same wire is bent in form of circle. Find the area of circle.
A horse is placed for grazing inside a rectangular field 40m by 36m and is tethered to one corner by a rope 14m long. Over how much area can it graze.
A sheet of paper is in the form of rectangle ABCD in which AB = 40cm and AD = 28 cm. A semicircular portion with BC as diameter is cut off. Find the area of remaining paper.
The circumference of two circles are in ratio 2:3. Find the ratio of their areas
The side of a square is 10 cm. find the area of circumscribed and inscribed circles.
The sum of the radii of two circles is 140 cm and the difference of their circumferences in 88 cm. Find the diameters of the circles.
The area of circle, inscribed in equilateral triangle is 154 cms2. Find the perimeter of
triangle.
A field is in the form of circle. A fence is to be erected around the field. The cost of fencing would to Rs. 2640 at rate of Rs.12 per metre. Then the field is to be thoroughs ploughed at cost of Rs. 0.50 per m2. What is amount required to plough the field?
If a square is inscribed in a circle, find the ratio of areas of the circle and the square.
A park is in the form of rectangle 120m × 100m. At the centre of park there is a circular lawn. The area of park excluding lawn is 8700m2. Find the radius of circular lawn.
The radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
The radii of two circles are 19cm and 9 cm respectively. Find the radius and area of the circle which has circumferences is equal to sum of circumference of two circles.
A car travels 1 km distance in which each wheel makes 450 complete revolutions. Find the radius of wheel.
The area enclosed between the concentric circles is 770cm2. If the radius of outer circle 21cm. find the radius of inner circle
An archery target has three regions formed by three concentric circles as shown in figure. If the diameters of the concentric circles are in the ratios 1 : 2 : 3, then find the ratio of the areas of three regions.
The wheel of a motor cycle is of radius 35 cm. How many revolutions per minute must the wheel make so as to keep a speed of 66 km/hr ?
A circular pond is 17.5 m is of diameter. It is surrounded by a 2 m wide path. Find the cost of constructing the path at the rate of Rs 25 per m2.
A circular park is surrounded by a road 21 m wide. If the radius of the park is 105 m, find the area of the road.
A square of diagonal 8 cm is inscribed in a circle. Find the area of the region lying outside the circle and inside the square.
Find the area enclosed between two concentric circles of radii 3.5 cm and 7 cm. A third concentric circle is drawn outside the 7 cm circle , such that the area enclosed between it and the 7 cm circle is same as that between the two inner circles . Find the radius of the third circle correct to one decimal place.
A path of width 3.5 m runs around a semi-circular grassy plot whose perimeter is 72 m . Find the area of the path .
`("Use" pi= 22/7) `
A circular pond is 17.5 m is of diameter. It is surrounded by a 2 m wide path. Find the cost of constructing the path at the rate of Rs 25 per m2.
The outer circumference of a circular race-track is 528 m . The track is everywhere 14 m wide. Calculate the cost of levelling the track at the rate of 50 paise per square metre.
`(use pi=22/7). `
A road which is 7 m wide surrounds a circular park whose circumference is 352 m . Find the area of the road .
Prove that the area of a circular path of uniform width h surrounding a circular region of radius r is `pih(2r+h)`
RD Sharma solutions for Mathematics [English] Class 10 13 Areas Related to Circles Exercise 13.2 [Pages 24 - 26]
Find in terms of x the length of the arc that subtends an angle of 30°, at the centre of circle of radius 4 cm.
Find the angle subtended at the centre of circle of radius 5cm by an arc of length `((5pi)/3)` cm
An arc of length 20𝜋 cm subtends an angle of 144° at centre of circle. Find the radius of the circle.
An arc of length 15 cm subtends an angle of 45° at the centre of a circle. Find in terms of 𝜋, radius of the circle.
Find the angle subtended at the centre of circle of radius ‘a’ cm by an arc of length
`(api)/4` 𝑐𝑚
A sector of circle of radius 4cm contains an angle of 30°. Find the area of sector
A sector of a circle of radius 8cm contains the angle of 135°. Find the area of sector.
The area of sector of circle of radius 2cm is 𝜋cm2. Find the angle contained by the sector.
The area of sector of circle of radius 5cm is 5𝜋 cm2. Find the angle contained by the sector.
AB is a chord of circle with centre O and radius 4cm. AB is length of 4cm. Find the area of sector of the circle formed by chord AB
In a circle of radius 35 cm, an arc subtends an angle of 72° at the centre. Find the length of arc and area of sector
The perimeter of a sector of circle of radius 5.7m is 27.2 m. Find the area of sector.
The perimeter of certain sector of circle of radius 5.6 m is 27.2 m. Find the area of sector.
A sector is cut-off from a circle of radius 21 cm the angle of sector is 120°. Find the length of its arc and its area.
The minute hand of a clock is √21 𝑐𝑚 long. Find area described by the minute hand on the face of clock between 7 am and 7:05 am
The minute hand of clock is10cm long. Find the area of the face of the clock described by the minute hand between 8am and 8:25 am
A sector of 56° cut out from a circle contains area of 4.4 cm2. Find the radius of the circle
Area of a sector of central angle 200° of a circle is 770 cm2. Find the length of the corresponding arc of this sector.
The length of the minute hand of a clock is 5 cm. Find the area swept by the minute hand during the time period 6 : 05 am and 6 : 40 am.
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes.
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the length of the arc. (Use π = `22/7`)
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the sector formed by the arc. (Use π = `22/7`)
From a circular piece of cardboard of radius 3 cm two sectors of 900 have been cutoff . Find the perimeter of the remaining portion nearest hundredth centimeters ( `"Take" pi = 22/ 7`).
The area of sector is one-twelfth that of the complete circle. Find the angle of the sector .
AB is a chord of a circle with centre O and radius 4 cm. AB is of length 4 cm. Find the area of the sector of the circle formed by chord AB.
In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find Circumference of the circle
In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find Area of the circle
In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find Length of arc
In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find The area of sector
Below fig shows a sector of a circle, centre O. containing an angle 𝜃°. Prove that
Area of shaded region is`r^2/2(tantheta −(pitheta)/180)`
Below fig shows a sector of a circle, centre O. containing an angle 𝜃°. Prove that Perimeter of shaded region is 𝑟 (tan 𝜃 + sec 𝜃 +`(pitheta)/180`− 1)
The diagram shows a sector of circle of radius ‘r’ can containing an angle 𝜃. The area of sector is A cm2 and perimeter of sector is 50 cm. Prove that
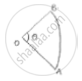
(i) 𝜃 =`360/pi(25/r− 1)`
(ii) A = 25r – r2
RD Sharma solutions for Mathematics [English] Class 10 13 Areas Related to Circles Exercise 13.3 [Pages 32 - 33]
AB is a chord of a circle with centre O and radius 4 cm. AB is of length 4 cm and divides the circle into two segments. Find the area of the minor segment.
A chord PQ of length 12 cm subtends an angle of 120° at the centre of a circle. Find the area of the minor segment cut off by the chord PQ.
A chord of circle of radius 14cm makes a right angle at the centre. Find the areas of minor and major segments of the circle.
A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the area of both
segments
A chord AB of circle, of radius 14cm makes an angle of 60° at the centre. Find the area of minor segment of circle.
Find the area of minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 600 .
A chord of a circle of radius 20 cm sub tends an angle of 900 at the centre . Find the area of the corresponding major segment of the circle
( Use \[\pi = 3 . 14\])
AB is the diameter of a circle, centre O. C is a point on the circumference such that ∠COB = 𝜃. The area of the minor segment cutoff by AC is equal to twice the area of sector BOC.Prove that `"sin"theta/2. "cos"theta/2= pi (1/2−theta/120^@)`
A chord of a circle subtends an angle 𝜃 at the centre of circle. The area of the minor segment cut off by the chord is one eighth of the area of circle. Prove that 8 sin`theta/2 "cos"theta/2+pi =(pitheta)/45`
RD Sharma solutions for Mathematics [English] Class 10 13 Areas Related to Circles Exercise 13.4 [Pages null - 65]
A plot is in the form of rectangle ABCD having semi-circle on BC. If AB = 60m and BC = 28m, find the area of plot.
A playground has the shape of rectangle, with two semicircles on its smaller sides as diameters, added to its outside. If the sides of rectangle are 36m and 24.5m. find the area of playground.
Find the area of the circle in which a square of area 64 cm2 is inscribed. [Use π = 3.14]
A rectangular piece is 20m long and 15m wide from its four corners, quadrants of 3.5m radius have been cut. Find the area of remaining part.
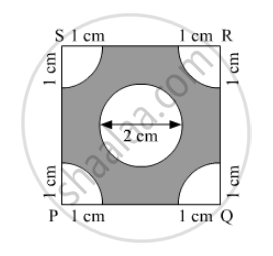
In the following figure, PQRS is a square of side 4 cm. Find the area of the shaded square.
Four cows are tethered at four corners of a square plot of side 50m, so that’ they just cant reach one another. What area will be left ungrazed.
A cow is tied with a rope of length 14 m at the corner of a rectangular field of dimensions 20 m × 16 m. Find the area of the field in which the cow can graze.
A calf is tied with a rope of length 6 m at the corner of a square grassy lawn of side 20 m . If the length of the rope is increased by 5.5 m , find the increase in area of the grassy lawn in which the calf can graze .
A square water tank has its side equal to 40 m. There are four semi-circular grassy plots all round it. Find the cost of turfing the plot at Rs. 1.25 per square metre (Take π = 3.14).
A rectangular park is 100 m by 50 m. It is surrounding by semi-circular flower beds all round. Find the cost of levelling the semi-circular flower beds at 60 paise per square metre (use π = 3.14).
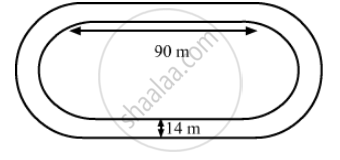
The inside perimeter of a running track (shown in the following figure) is 400 m. The length of each of the straight portion is 90 m and the ends are semi-circles. If the track is everywhere 14 m wide. find the area of the track. Also find the length of the outer running track.
Find the area of the following figure, in square cm, correct to one place of decimal. (Take π = 22/7).
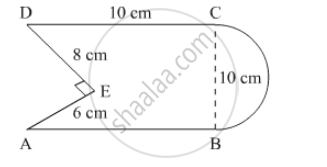
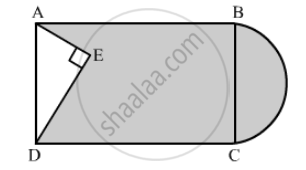
In the following figure, from a rectangular region ABCD with AB = 20 cm, a right triangle AED with AE = 9 cm and DE = 12 cm, is cut off. On the other end, taking BC as diameter, a semicircle is added on outside' the region. Find the area of the shaded region. [Use π =]`22/7` [CBSE 2014]
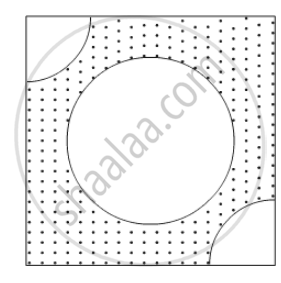
From each of the two opposite corners of a square of side 8 cm, a quadrant of a circle of radius 1.4 cm is cut. Another circle of radius 4.2 cm is also cut from the centre as shown in the following figure. Find the area of the remaining (Shaded) portion of the square. (Use π = 22/7)
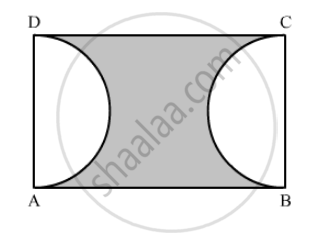
In the following figure, ABCD is a rectangle with AB = 14 cm and BC = 7 cm. Taking DC, BC and AD as diameters, three semi-circles are drawn as shown in the figure. Find the area of the shaded region.
In the following figure, ABCD is a rectangle, having AB = 20 cm and BC = 14 cm. Two sectors of 180° have been cut off. Calculate:
the area of the shaded region.
In the following figure, ABCD is a rectangle, having AB = 20 cm and BC = 14 cm. Two sectors of 180° have been cut off. Calculate:
the length of the boundary of the shaded region.
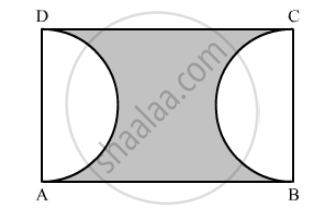
In the following figure, the square ABCD is divided into five equal parts, all having same area. The central part is circular and the ines AE, GC, BF and HD lie along the diagonals AC and BD of the square. If AB = 22 cm, find:
the circumference of the central part.

In the following figure, the square ABCD is divided into five equal parts, all having same area. The central part is circular and the lines AE, GC, BF and HD lie along the diagonals AC and BD of the square. If AB = 22 cm, find:
the perimeter of the part ABEF.

In the following figure find the area of the shaded region. (Use π = 3.14)
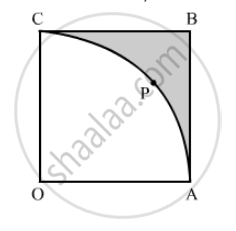
In the following figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the (i) quadrant OACB (ii) shaded region.

In the following figure a square OABC is inscribed in a quadrant OPBQ of a circle. If OA = 21 cm, find the area of the shaded region.

In the following figure, OABC is a square of side 7 cm. If OAPC is a quadrant of a circle with centre O, then find the area of the shaded region. (Use π = 22/7)
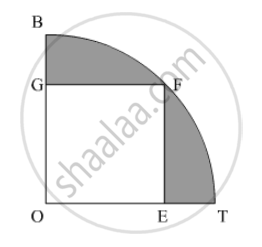
In the following figure, OE = 20 cm. In sector OSFT, square OEFG is inscribed. Find the area of the shaded region.
Find the area of the shaded region in the following figure, if AC = 24 cm, BC = 10 cm and O is the centre of the circle. (Use π = 3.14)

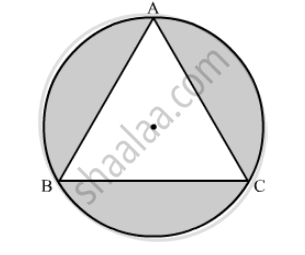
A circle is inscribed in an equilateral triangle ABC is side 12 cm, touching its sides (the following figure). Find the radius of the inscribed circle and the area of the shaded part.

In the following figure, an equilateral triangle ABC of side 6 cm has been inscribed in a circle. Find the area of the shaded region. (Take π = 3.14).
A circular field has a perimeter of 650 m. A square plot having its vertices on the circumference of the field is marked in the field. Calculate the area of the square plot.
Find the area of a shaded region in the the following figure,where a circular arc of radius 7 cm has been drawn with vertex A of an equilateral triangle ABC of side 14 cm as centre. (Use π = 22/7 and \[\sqrt{3}\] = 1.73)

A regular hexagon is inscribed in a circle. If the area of hexagon is \[24\sqrt{3}\] , find the area of the circle. (Use π = 3.14)
ABCDEF is a regular hexagon with centre O (in the following figure). If the area of triangle OAB is 9 cm2, find the area of : (i) the hexagon and (ii) the circle in which the haxagon is incribed.
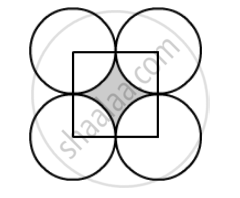
Four equal circles, each of radius 5 cm touch each other as shown in fig. Find the area included etween them.
Four equal circles each of radius a, touch each other. Show that area between them is `6/7a^2`
A child makes a poster on a chart paper drawing a square ABCD of side 14 cm. She draws four circles with centre A, B, C and D in which she suggests different ways to save energy. The circles are drawn in such a way that each circle touches externally two of the three remaining circles (in the following figure). In the shaded region she write a message 'Save Energy'. Find the perimeter and area of the shaded region.
(Use π = 22/7)

The diameter of a coin is 1 cm (in the following figure). If four such coins be placed on a table so that the rim of each touches that of the other two, find the area of the shaded region (Take π = 3.1416).
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm × 7 cm. Find the area of the remaining card board. (Use π = 22/7).
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm × 7 cm. Find the area of the remaining card board. (Use π = 22/7).
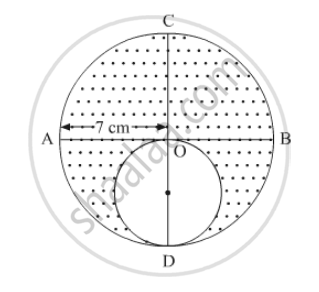
In the following figure, AB and CD are two diameters of a circle perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.
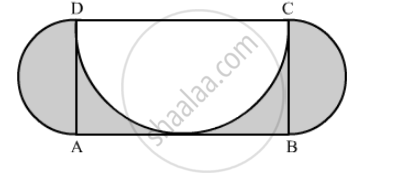
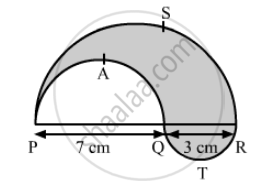
In the the following figure, PSR, RTQ and PAQ are three semicircles of diameter 10 cm, 3 cm and 7 cm respectively. Find the perimeter of shaded region.
In the following figure, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.
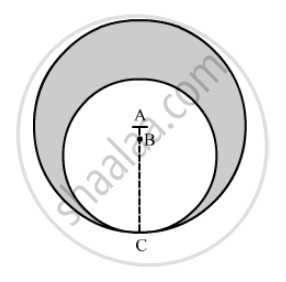
In the following figure, ABCD is a square of side 2a, Find the ratio between
(i) the circumferences
(ii) the areas of the in circle and the circum-circle of the square.
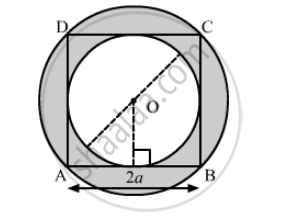
In the following figure, there are three semicircles, A, B and C having diameter 3 cm each, and another semicircle E having a circle D with diameter 4.5 cm are shown. Calculate:
(i) the area of the shaded region
(ii) the cost of painting the shaded region at the rate of 25 paise per cm2 , to the nearest rupee.

In the following figure, ABC is a right-angled triangle, ∠B = 90°, AB = 28 cm and BC = 21 cm. With AC as diameter a semicircle is drawn and with BC as radius a quarter circle is drawn. Find the area of the shaded region correct to two decimal places.
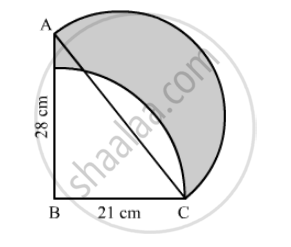
In the following figure, O is the centre of a circular arc and AOB is a straight line. Find the perimeter and the area of the shaded region correct to one decimal place. (Take π = 3.142)
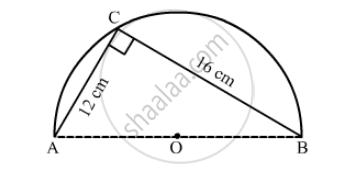
In the following figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
the length of the boundary.
the area of the shaded region.

In the following figure, AB = 36 cm and M is mid-point of AB. Semi-circles are drawn on AB, AM and MB as diameters. A circle with centre C touches all the three circles. Find the area of the shaded region.

In the following figure, ABC is a right angled triangle in which ∠A = 90°, AB = 21 cm and AC = 28 cm. Semi-circles are described on AB, BC and AC as diameters. Find the area of the shaded region.
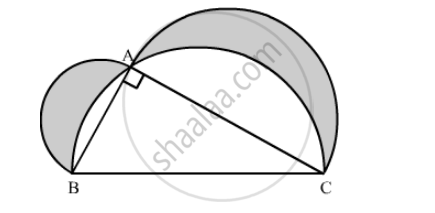
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the height of the tunnel
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the perimeter of the cross-section
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the area of the cross-section.
In the following figure, shows a kite in which BCD is the shape of a quadrant of a circle of radius 42 cm. ABCD is a square and Δ CEF is an isosceles right angled triangle whose equal sides are 6 cm long. Find the area of the shaded region.

In the following figure, ABCD is a trapezium of area 24.5 cm2 , If AD || BC, ∠DAB = 90°, AD = 10 cm, BC = 4 cm and ABE is quadrant of a circle, then find the area of the shaded region. [CBSE 2014]

In the given figure, ABCD is a trapezium with AB || DC, AB = 18 cm DC = 32 cm and the distance between AB and DC is 14 cm. Circles of equal radii 7 cm with centres A, B, C and D have been drawn. Then find the area of the shaded region.
(Use \[\pi = \frac{22}{7}\]
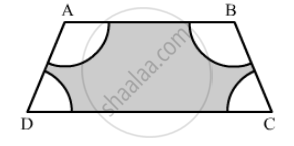
From a thin metallic piece, in the shape of a trapezium ABCD, in which AB || CD and ∠BCD = 90°, a quarter circle BEFC is removed (in the following figure). Given AB = BC = 3.5 cm and DE = 2 cm, calculate the area of the remaining piece of the metal sheet.
In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

Sides of a triangular field are 15 m, 16 m and 17 m. With the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7 m each to graze in the field. Find the area of the field which cannot be grazed by the three animals.
In the given figure, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where O and O' are centres of the circles. Find the area of shaded region.

In a hospital used water is collected in a cylindrical tank of diameter 2 m and height 5 m. After recycling, this water is used to irrigate a park of hospital whose length is 25 m and breadth is 20 m. If tank is filled completely then what will be the height of standing water used for irrigating the park.
RD Sharma solutions for Mathematics [English] Class 10 13 Areas Related to Circles Exercise 13.5 [Pages 67 - 68]
What is the ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal?
If the circumference of two circles are in the ratio 2 : 3, what is the ratio of their areas?
Write the area of the sector of a circle whose radius is r and length of the arc is l.
What is the length (in terms of π) of the arc that subtends an angle of 36° at the centre of a circle of radius 5 cm?
What is the angle subtended at the centre of a circle of radius 6 cm by an arc of length 3 π cm?
What is the area of a sector of a circle of radius 5 cm formed by an arc of length 3.5 cm?
In a circle of radius 10 cm, an arc subtends an angle of 108° at the centre. what is the area of the sector in terms of π?
If a square is inscribed in a circle, what is the ratio of the areas of the circle and the square?
Write the formula for the area of a sector of angle \[\theta\] (in degrees) of a circle of radius r.
Write the formula for the area of a segment in a circle of radius r given that the sector angle is \[\theta\] (in degrees).
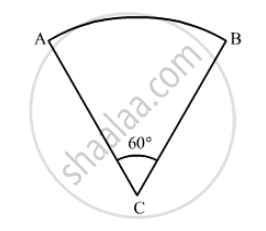
If the adjoining figure is a sector of a circle of radius 10.5 cm, what is the perimeter of the sector? (Take \[\pi = 22/7\])
If the diameter of a semi-circular protractor is 14 cm, then find its perimeter.
An arc subtends an angle of 90° at the centre of the circle of the radius 14 cm. Write the area of minor sector thus formed in terms of π.
Find the area of the largest triangle that can be inscribed in a semi-circle of radius runits.
Find the area of sector of circle of radius 21 cm and central angle 1200.
What is the area of a square inscribed in a circle of diameter p cm ?
If the numerical value of the area of a circle is equal to the numerical value of its circumference , find its radius.
How many revolutions a circular wheel of radius r metres makes in covering a distance of s metres?
Find the ratio of the area of the circle circumscribing a square to the area of the circle inscribed in the square .
RD Sharma solutions for Mathematics [English] Class 10 13 Areas Related to Circles Exercise 13.6 [Pages 69 - 74]
If the circumference and the area of a circle are numerically equal, then diameter of the circle is
\[\frac{\pi}{2}\]
2\[\frac{\pi}{2}\]
2
4
If the difference between the circumference and radius of a circle is 37 cm, then using π = \[\frac{22}{7}\] the circumference (in cm) of the circle is
154
44
14
7
A wire can be bent in the form of a circle of radius 56 cm. If it is bent in the form fo a square, then its area will be
3520 cm2
6400 cm2
7744 cm2
8800 cm2
If a wire is bent into the shape of a square, then the area of the square is 81 cm2. When wire is bent into a semi-circular shape, then the area of the semi-circle will be ______.
22 cm2
44 cm2
77 cm2
154 cm2
A circular park has a path of uniform width around it. The difference between the outer and inner circumferences of the circular path is 132 m. Its width is
20 m
21 m
22 m
24 m
The radius of a wheel is 0.25 m. The number of revolutions it will make to travel a distance of 11 km will be
2800
4000
5500
7000
The ratio of the outer and inner perimeters of a circular path is 23 : 22. If the path is 5 metres wide, the diameter of the inner circle is
55 m
110 m
220 m
230 m
The circumference of a circle is 100 m. The side of a square inscribed in the circle is
`50sqrt2`
`50/π`
`(50sqrt(2))/π`
`(100sqrt(2))/π`
The area of the incircle of an equilateral triangle of side 42 cm is
\[22\sqrt{3} c m^2\]
231 cm2
462 cm2
924 cm2
The area of incircle of an equilateral triangle is 154 cm2 . The perimeter of the triangle is
71.5 cm
71.7 cm
72.3 cm
72.7 cm
The area of the largest triangle that can be inscribed in a semi-circle of radius r, is
r2
2r2
r3
2r3
The perimeter of a triangle is 30 cm and the circumference of its incircle is 88 cm. The area of the triangle is
70 cm2
140 cm2
210 cm2
420 cm2
The area of a circle is 220 cm2. The area of ta square inscribed in it is
49 cm2
70 cm2
140 cm2
150 cm2
If the circumference of a circle increases from 4π to 8π, then its area is
halved
doubled
tripled
quadrupled
If the radius of a circle is diminished by 10%, then its area is diminished by
10%
19%
20%
36%
If the area of a square is same as the area of a circle, then the ratio of their perimeters, in terms of π, is
π :\[\sqrt{3}\]
2 : \[\sqrt{\pi}\]
3 :\[\pi\]
\[\pi : \sqrt{2}\]
The area of the largest triangle that can be inscribed in a semi-circle of radius r is
2r
r2
r
\[\sqrt{r}\]
The ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal, is
\[\pi: \sqrt{2}\]
\[\pi: \sqrt{3}\]
\[\sqrt{3}: \pi\]
\[\sqrt{2}: \pi\]
If the sum of the areas of two circles with radii r1 and r2 is equal to the area of a circle of radius r, then \[r_1^2 + r_2^2\]
>r2
=r2
<r2
None of these
If the perimeter of a semi-circular protractor is 36 cm, then its diameter is
10 cm
12 cm
14 cm
16 cm
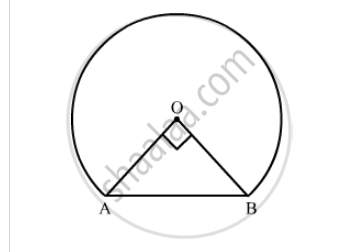
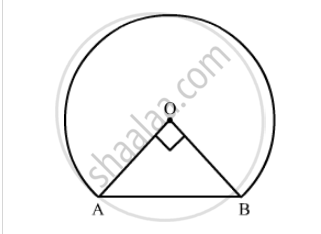
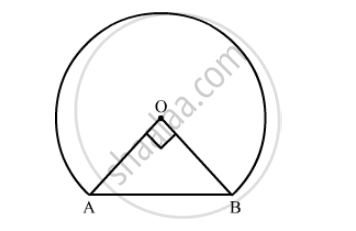
The perimeter of the sector OAB shown in the following figure, is

\[\frac{64}{3} cm\]
26 cm
\[\frac{64}{5} cm\]
19 cm
If the perimeter of a sector of a circle of radius 6.5 cm is 29 cm, then its area is
58 cm2
52 cm2
25 cm2
56 cm2
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then its radius is
12 cm
16 cm
8 cm
10 cm
The area of the circle that can be inscribed in a square of side 10 cm is
40 π cm2
30 π cm2
100 π cm2
25 π cm2
If the difference between the circumference and radius of a circle is 37 cm, then its area is
154 cm2
160 cm2
200 cm2
150 cm2
The area of a circular path of uniform width h surrounding a circular region of radius r is
\[\pi(2r + h)r\]
\[\pi(2r + h)r\]
\[\pi(2r + h)h\]
\[\pi(h + r)r\]
\[\pi(h + r)h\]
If AB is a chord of length \[5\sqrt{3}\] cm of a circle with centre O and radius 5 cm, then area of sector OAB is
\[\frac{3\pi}{8}c m^2 \]
\[\frac{8\pi}{3}c m^2\]
\[25 \pi cm^2\]
\[\frac{25\pi}{3}c m^2\]
The area of a circle whose area and circumference are numerically equal, is
2 \[\pi\] sq. units
4 \[\pi\] units
6\[\pi\]sq. units
8 \[\pi\] sq. units
If diameter of a circle is increased by 40%, then its area increase by
96%
40%
80%
48%
In the following figure, the shaded area is

50 (π−2) cm2
25 (π−2) cm2
25 (π+2) cm2
5 (π−2) cm2
In the following figure, the area of the segment PAQ is

\[\frac{a^2}{4}\left( \pi + 2 \right)\]
\[\frac{a^2}{4}\left( \pi - 2 \right)\]
\[\frac{a^2}{4}\left( \pi - 1 \right)\]
\[\frac{a^2}{4}\left( \pi + 1 \right)\]
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then the radius of the circle
12 cm
16 cm
8 cm
10 cm
If the area of a sector of a circle is `5/18` of the area of the circle, then the sector angle is equal to
60°
90°
100°
120°
If he area of a sector of a circle is \[\frac{7}{20}\] of the area of the circle, then the sector angle is equal to
110°
130°
100°
126°
In the following figure, If ABC is an equilateral triangle, then shaded area is equal to
\[\left( \frac{\pi}{3} - \frac{\sqrt{3}}{4} \right) r^2\]
\[\left( \frac{\pi}{3} - \frac{\sqrt{3}}{2} \right) r^2\]
\[\left( \frac{\pi}{3} + \frac{\sqrt{3}}{4} \right) r^2\]
\[\left( \frac{\pi}{3} + \sqrt{3} \right) r^2\]
In the following figure, the area of the shaded region is
3π cm2
6π cm2
9π cm2
7π cm2
If the perimeter of a circle is equal to that of a square, then the ratio of their areas is ______.
22 : 7
13 : 22
14 : 11
7 : 22
22 : 13
11 : 14
The radius of a circle is 20 cm. It is divided into four parts of equal area by drawing three concentric circles inside it. Then, the radius of the largest of three concentric circles drawn is
10 \[\sqrt{5}\]
10\[\sqrt{3}\]cm
10\[\sqrt{5}\]
10\[\sqrt{2}\]
The area of a sector whose perimeter is four times its radius r units, is
\[\frac{r^2}{4}\]
2r2 sq. units
r2 sq.units
If a chord of a circle of radius 28 cm makes an angle of 90 ° at the centre, then the area of the major segment is
392 cm2
1456 cm2
1848 cm2
2240 cm2
If area of a circle inscribed in an equilateral triangle is 48π square units, then perimeter of the triangle is
17 \[\sqrt{3}\]units
36 units
72 units
48\[\sqrt{3}\]units
The hour hand of a clock is 6 cm long. The area swept by it between 11.20 am and 11.55 am is
2.75 cm2
5.5 cm2
11 cm2
10 cm2
ABCD is a square of side 4 cm. If E is a point in the interior of the square such that ΔCEDis equilateral, then area of Δ ACE is
\[2\sqrt{3} - 1 c m^2\]
\[4\sqrt{3} - 1 c m^2\]
\[6\sqrt{3} - 1 c m^2\]
\[8\sqrt{3} - 1 c m^2\]
If the area of a circle is equal to the sum of the areas of two circles of diameters 10 cm and 24 cm, then diameter of the large circle (in cm) is
34
26
17
14
If π is taken as `22/7` the distance (in metres) covered by a wheel of diameter 35 cm, in one revolution, is
2.2
1.1
9.625
96.25
ABCD is a rectangle whose three vertices are B (4,0), C (4,3) and D (0, 3). The length of one of its diagonals is
5
4
3
25
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
r2 sq.units
r2
2r2
`1/2 "r"^2` sq.units
2r2 sq.units
r3
`sqrt(2)"r"^2` sq.units
2r3
If the sum of the areas of two circles with radii R1 and R2 is equal to the area of a circle of radius R, then ______.
R1 + R2 = R
`"R"_1^2 + "R"_2^2 = "R"^2`
R1 + R2 < R
`"R"_1^2 + "R"_2^2 < "R"^2`
If the sum of the circumferences of two circles with radii R1 and R2 is equal to the circumference of a circle of radius R, then ______.
R1 + R2 = R
R1 + R2 > R
R1 + R2 < R
Nothing definite can be said about the relation among R1, R2 and R.
None of these
If the circumference of a circle and the perimeter of a square are equal, then ______.
Area of the circle = Area of the square
Area of the circle > Area of the square
Area of the circle < Area of the square
Nothing definite can be said about the relation between the areas of the circle and square.
None of these
If the perimeter of a circle is equal to that of a square, then the ratio of their areas is ______.
22 : 7
13 : 22
14 : 11
7 : 22
22 : 13
11 : 14
Solutions for 13: Areas Related to Circles
![RD Sharma solutions for Mathematics [English] Class 10 chapter 13 - Areas Related to Circles RD Sharma solutions for Mathematics [English] Class 10 chapter 13 - Areas Related to Circles - Shaalaa.com](/images/8193647920-mathematics-english-class-10_6:5809898a5fef45e9a2f7e6b414d392fa.jpg)
RD Sharma solutions for Mathematics [English] Class 10 chapter 13 - Areas Related to Circles
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 10 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. RD Sharma solutions for Mathematics Mathematics [English] Class 10 CBSE 13 (Areas Related to Circles) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. RD Sharma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 10 chapter 13 Areas Related to Circles are Areas of Combinations of Plane Figures, Circumference of a Circle, Perimeter and Area of a Circle - A Review, Areas of Sector and Segment of a Circle, Area of Circle, Circles Passing Through One, Two, Three Points, Converse of Tangent Theorem, Tangent Segment Theorem, Inscribed Angle Theorem, Corollaries of Inscribed Angle Theorem, Theorem: Opposite angles of a cyclic quadrilateral are supplementary., Corollary of Cyclic Quadrilateral Theorem, Converse: If a Pair of Opposite Angles of a Quadrilateral is Supplementary, Then the Quadrilateral is Cyclic., Theorem of Angle Between Tangent and Secant, Converse of Theorem of the Angle Between Tangent and Secant, Theorem of Internal Division of Chords, Theorem of External Division of Chords, Tangent to a Circle, Number of Tangents from a Point on a Circle, Touching Circles, Concept of Circle, Secant and Tangent, Theorem of Touching Circles, Tangent Properties - If Two Circles Touch, the Point of Contact Lies on the Straight Line Joining Their Centers, Introduction to an Arc, Congruence of Arcs, Property of Sum of Measures of Arcs, Inscribed Angle, Intercepted Arc, Cyclic Quadrilateral, Converse of Cyclic Quadrilateral Theorem, Tangent Secant Segments Theorem, Tangent - Secant Theorem, Angle Subtended by the Arc to the Point on the Circle, Angle Subtended by the Arc to the Centre.
Using RD Sharma Mathematics [English] Class 10 solutions Areas Related to Circles exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in RD Sharma Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 10 students prefer RD Sharma Textbook Solutions to score more in exams.
Get the free view of Chapter 13, Areas Related to Circles Mathematics [English] Class 10 additional questions for Mathematics Mathematics [English] Class 10 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
