Advertisements
Advertisements
प्रश्न
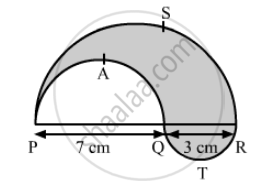
In the the following figure, PSR, RTQ and PAQ are three semicircles of diameter 10 cm, 3 cm and 7 cm respectively. Find the perimeter of shaded region.
उत्तर
Perimeter of shaded region = Length of the arc PAQ + Length of the arc PSR + Length of the arc RTQ
\[= \frac{1}{2} \times 2\pi r_1 + \frac{1}{2} \times 2\pi r_2 + \frac{1}{2} \times 2\pi r_3 \]
\[ = \frac{1}{2} \times 2\pi\left( \frac{7}{2} \right) + \frac{1}{2} \times 2\pi\left( \frac{10}{2} \right) + \frac{1}{2} \times 2\pi\left( \frac{3}{2} \right)\]
\[ = \frac{7}{2}\pi + 5\pi + \frac{3}{2}\pi\]
\[ = 10\pi\]
\[ = 31 . 4 cm\]
Hence, the perimeter of shaded region is 31.4 cm.
APPEARS IN
संबंधित प्रश्न
A chord PQ of length 12 cm subtends an angle of 120° at the centre of a circle. Find the area of the minor segment cut off by the chord PQ.
A chord of a circle of radius 20 cm sub tends an angle of 900 at the centre . Find the area of the corresponding major segment of the circle
( Use \[\pi = 3 . 14\])
A circle is inscribed in an equilateral triangle ABC is side 12 cm, touching its sides (the following figure). Find the radius of the inscribed circle and the area of the shaded part.

In the given figure, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where O and O' are centres of the circles. Find the area of shaded region.

If the numerical value of the area of a circle is equal to the numerical value of its circumference , find its radius.
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then its radius is
The area of a circle whose area and circumference are numerically equal, is
A square is inscribed in a circle. Find the ratio of the areas of the circle and the square.
The area of a circle is 98.56 cm2. Find its circumference.
Area of circle of radius ‘n’ units is
