Advertisements
Advertisements
प्रश्न
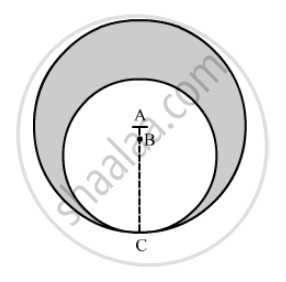
In the following figure, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.
उत्तर
Area of the shaded region can be calculated as shown below,
Area of the shaded region = Area of circle with radius AC − area of circle with radius BC
We have given radius of the outer circle that is 8 cm but we don’t know the radius of the inner circle.
We can calculate the radius of the inner circle as shown below,
`BC=AC-AB`
`∴ BC=8-3`
`∴ BC=5`
`∴" Area of the shaded region"=pixx8xx8-pixx5xx5`
`∴" Area of the shaded region"=pixx64-pixx25`
`∴" Area of the shaded region"=pixx39`
Substituting `pi=22/7` we get`
`∴" Area of the shaded region"=22/7xx39`
`∴" Area of the shaded region"=122.57`
Therefore, area of the shaded region is `122.57 cm^2`
APPEARS IN
संबंधित प्रश्न
From a circular piece of cardboard of radius 3 cm two sectors of 900 have been cutoff . Find the perimeter of the remaining portion nearest hundredth centimeters ( `"Take" pi = 22/ 7`).
A circle is inscribed in an equilateral triangle ABC is side 12 cm, touching its sides (the following figure). Find the radius of the inscribed circle and the area of the shaded part.

A circular park has a path of uniform width around it. The difference between the outer and inner circumferences of the circular path is 132 m. Its width is
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then the radius of the circle
The radius of a circle is 20 cm. It is divided into four parts of equal area by drawing three concentric circles inside it. Then, the radius of the largest of three concentric circles drawn is
A square is inscribed in a circle. Find the ratio of the areas of the circle and the square.
On increasing the diameter of a circle by 40%, its area will be increased by
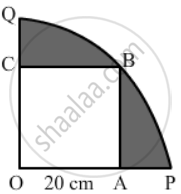
In the given figure, a square OABC has been inscribed in the quadrant OPBQ. If OA = 20 cm, then the area of the shaded region is
A circular field of radius 105 m has a circular path of uniform width of 5 m along and inside its boundary. Find the area of the path.
Area of a circle with diameter ‘m’ radius ‘n’ and circumference ‘p’ is ______.
