Advertisements
Advertisements
प्रश्न
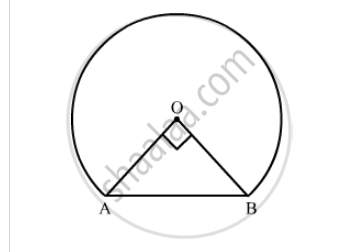
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the height of the tunnel
उत्तर
We have a cross section of a railway tunnel. `ΔOAB`is a right angled isosceles triangle, right angled at O. let OM be perpendicular to AB.
`OA=2 m`
Use Pythagoras theorem in `ΔOAB`to get,
`AB=(sqrt(2^2+2^2))m`
`= 2sqrt2 m`
Let the height of the tunnel be h. So,
`"Area of" ΔOAB=1/2(2)(2)`
`1/2(2sqrt2)(OM)=2`
Thus,
`OM=sqrt2m`
Therefore,
`h=(2+sqrt2)m`
APPEARS IN
संबंधित प्रश्न
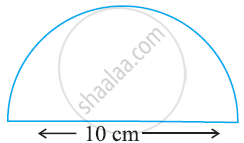
Find the perimeter of the adjoining figure, which is a semicircle including its diameter.
A carpet is laid on floor of a room 8 m by 5 m. There is border of constant width all around the carpet. If the area of the border is `12m^2` find its width.
The cost of harvesting a square field at ₹ 900 per hectare is ₹ 8100. Find the cost of putting a fence around it at ₹ 18 per meter.
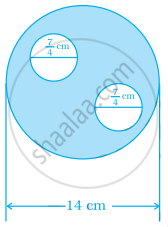
In the following figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
the length of the boundary.
the area of the shaded region.

If the circumference of a circle increases from 4π to 8π, then its area is
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then its radius is
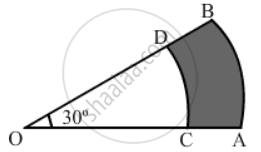
In the given figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.
The minute hand of a clock is 8 cm long. Find the area swept by the minute hand between 8.30 a.m. and 9.05 a.m.
The formula used to find the area of the circle is ________ sq.units
Find the area of the shaded region: