Advertisements
Advertisements
प्रश्न
In the given figure, find the area of ΔGEF.
उत्तर
Given: (1) ABCD is a rectangle.
(2) CD = 6 cm
(3) AD = 8cm
To find: Area of ΔGEF.
Calculation: We know that,
Area of Parallelogram = base × height
If a triangle and a parallelogram are on the same base and between the same parallels , the area of the triangle is equal to half of the parallelogram
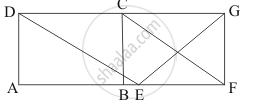
Here we can see that Parallelogram ABCD and triangle GEF are between the same base and same parallels.
Hence,
Area of ΔGEF = `1/2` Area of Parallelogram ABCD
`= 1/2 xx AD xx CD `
`= 1/2 xx 8 xx6`
= 24 cm2
Hence we get the result as Area of ΔGEF = 24 cm2
APPEARS IN
संबंधित प्रश्न
If P is any point in the interior of a parallelogram ABCD, then prove that area of the
triangle APB is less than half the area of parallelogram.
If ABC and BDE are two equilateral triangles such that D is the mid-point of BC, then find ar (ΔABC) : ar (ΔBDE).
ABCD is a parallelogram. P is any point on CD. If ar (ΔDPA) = 15 cm2 and ar (ΔAPC) = 20 cm2, then ar (ΔAPB) =
The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is ______.
The length and breadth of a rectangular piece of land are in the ratio 5 : 3. If the total cost of fencing it at the rate of ₹24 per meter is ₹9600, find its :
(i) length and breadth
(ii) area
(iii) cost of levelling at the rate of ₹60 per m2.
Find the area of a rectangle whose length = 8.5 m breadth = 5 m.
Find the area of a rectangle whose length = 24 cm breadth =180 mm
The side of a square field is 16 m. What will be increase in its area, if each of its sides is increased by 4 m?
By counting squares, estimate the area of the figure.

Find the area of the following figure by counting squares:

