Advertisements
Advertisements
प्रश्न
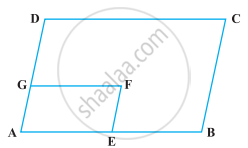
In the Figure, ABCD is a rectangle and EFGH is a parallelogram. Using the measurements given in the figure, what is the length d of the segment that is perpendicular to `bar("HE")` and `bar("FG")`?
उत्तर
In the given figure ABCD is a rectangle and EFGH is a parallelogram.
In the right triangle AEH
HE = `sqrt("AH"^2 + "AE"^2)`
= `sqrt(3^2 + 4^2)`
= `sqrt(9 + 16)`
= `sqrt(25)`
HE = 5
∴ GF = 5 ...(HE and Gf are opposite sides of a parallelogram)
In the right triangle
GC = `sqrt("GF"^2 - "FC"^2)`
= `sqrt(5^2 - 3^2)`
= `sqrt(25 - 9)`
= `sqrt(16)`
∴ DG = 10 – 6 = 4
Area of ΔAEH + Area of ΔBEF + Area of ΔFCG + Area of ΔHDG
= `1/2 xx 3 xx 4 + 1/2 xx 6 xx 5 + 1/2 xx 3 xx 4 + 1/2 xx 5 xx 6`
= (6 + 15 + 6 + 15)
= 42
∴ Area of 4 triangles = 42
Area of the parallelogram = Area of the rectangle ABCD – Area of 4 triangles.
= 10 × 8 – 42
= 80 – 42
= 38
b × h = 38
5 × d = 38
d = `38/5`
= `7 3/5`
Length of d = `7 3/5` or 7.6
APPEARS IN
संबंधित प्रश्न
The diagonal BD of a parallelogram ABCD bisects angles B and D. Prove that ABCD is a rhombus.
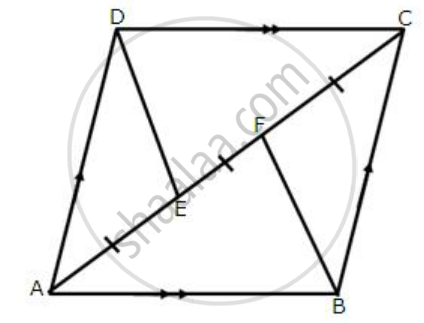
The alongside figure shows a parallelogram ABCD in which AE = EF = FC.
Prove that:
- DE is parallel to FB
- DE = FB
- DEBF is a parallelogram.
In parallelogram ABCD, the bisector of angle A meets DC at P and AB = 2 AD.
Prove that:
(i) BP bisects angle B.
(ii) Angle APB = 90o.
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: RT bisects angle R
ABCD is a parallelogram. The bisector of ∠BAD meets DC at P, and AD is half of AB.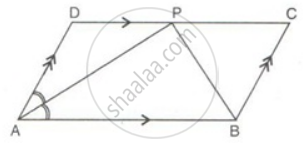
Prove that: BP bisects ∠ABC.
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that: BC = BE.
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that:CE is the bisector of angle C and angle DEC is a right angle
In the given figure, the perimeter of parallelogram PQRS is 42 cm. Find the lengths of PQ and PS.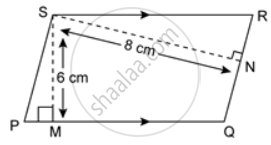
In parallelogram ABCD of the accompanying diagram, line DP is drawn bisecting BC at N and meeting AB (extended) at P. From vertex C, line CQ is drawn bisecting side AD at M and meeting AB (extended) at Q. Lines DP and CQ meet at O. Show that the area of triangle QPO is `9/8` of the area of the parallelogram ABCD
In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.