Advertisements
Advertisements
प्रश्न
In the following figure, AD is the bisector of ∠BAC. Prove that AB > BD.

उत्तर
Given: ABC is a triangle such that AD is the bisector of ∠BAC.
To prove: AB > BD.
Proof: Since, AD is the bisector of ∠BAC.
But ∠BAD = CAD ...(i)
∴ ∠ADB > ∠CAD ...[Exterior angle of a triangle is greater than each of the opposite interior angle]
∴ ∠ADB > ∠BAD ...[From equation (i)]
AB > BD ...[Side opposite to greater angle is longer]
Hence proved.
APPEARS IN
संबंधित प्रश्न
Can a triangle have two acute angles?Justify your answer in case.
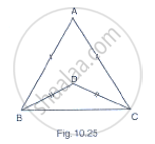
In Fig. 10.25, AB = AC and DB = DC, find the ratio ∠ABD : ∠ACD.
Is the following statement true and false :
A triangle can have two obtuse angles.
Is the following statement true and false :
An exterior angle of a triangle is greater than the opposite interior angles.
If one angle of a triangle is equal to the sum of the other two angles, then the triangle is
In a triangle PQR, ∠P = 60° and ∠Q = ∠R, find ∠R.
The length of the three segments is given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
17 cm, 7 cm, 8 cm
In ΔABC, name the 
a) Three sides: _________, __________, __________
b) Three Angles: _________, __________, __________
c) Three Vertices: _________, __________, __________
Can we have two acute angles whose sum is a reflex angle? Why or why not?
Jincy used these shapes to make drawings of fish.

Now you also use some shapes to draw the different sea animals shown below.

Sea urchin

Lobster

Eel

Red snapper

Octopus

Clam

Parrotfish

Prawn

Cuttlefish

Jellyfish

Squid

Silver pomfret

Crab
