Question

In the given diagram, OA = OB, ∠OAB = 𝜃 and the line AB passes through point P (-3, 4).

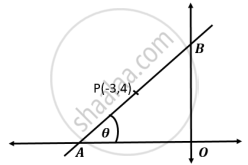
Find:

1. Slope and inclination (𝜃) of the line AB
2. Equation of the line AB

Answer 1

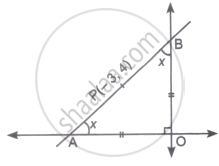
OA = OB

Given, Isosceles Δ so, ∠BAO = ∠ABO = x (let)

∠BAO + ∠ABO + ∠AOB = 180°     ...(∵ sum of three angles of a triangle is 180°)

⇒ x + x + 90° = 180°

⇒ 2x = 90°

⇒ x =  45°

∴ ∠BAO = 45° = 𝜃

(a) Slope of AB = tan 45° = 1

Inclination (𝜃) = 45°

(b) AB has slope = 1 and passes through P (-3, 4)

∴ Equation of the line AB is

⇒ y - y₁ = m(x - x₁)

⇒ y - 4 = 1(x + 3)

⇒ y = x + 3 + 4

y = x + 7

⇒ x - y + 7 = 0