Advertisements
Advertisements
प्रश्न
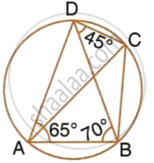
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find:
- ∠BCD
- ∠ACB
Hence, show that AC is a diameter.
उत्तर

i. In cyclic quadrilateral ABCD,
∠BCD = 180° – ∠BAD
= 180° – 65°
= 115°
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
ii. By angle sum property of ∆ABD,
∠ADB = 180° – 65° – 70° = 45°
Again, ∠ACB = ∠ADB = 45°
(Angle in the same segment)
∴ ∠ADC = ∠ADB + ∠BDC
= 45° + 45°
= 90°
Hence, AC is a semicircle.
(Since angle in a semicircle is a right angle)
APPEARS IN
संबंधित प्रश्न
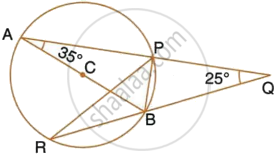
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find : ∠PRB
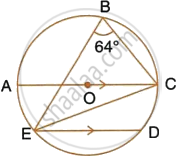
In the given figure, AOC is a diameter and AC is parallel to ED. If ∠CBE = 64°, calculate ∠DEC.
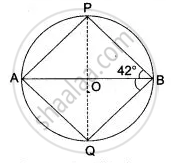
The given figure shows a circle with centre O and ∠ABP = 42°.

Calculate the measure of:
- ∠PQB
- ∠QPB + ∠PBQ
In the given circle with diameter AB, find the value of x.
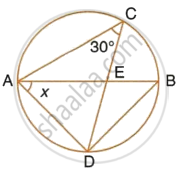
In cyclic quadrilateral ABCD; AD = BC, ∠BAC = 30° and ∠CBD = 70°; find:
- ∠BCD
- ∠BCA
- ∠ABC
- ∠ADC
In the given figure, ∠ACE = 43° and ∠CAF = 62°; find the values of a, b and c.

AB is a diameter of the circle APBR, as shown in the figure. APQ and RBQ are straight lines. Find : ∠BPR
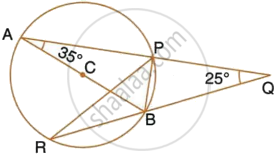
In the following figure, O is the centre of the circle, ∠ PBA = 42°.
Calculate:
(i) ∠ APB
(ii) ∠PQB
(iii) ∠ AQB
In the given figure, ∠CAB = 25°, find ∠BDC, ∠DBA and ∠COB
In the given figure (drawn not to scale) chords AD and BC intersect at P, where AB = 9 cm, PB = 3 cm and PD = 2 cm.
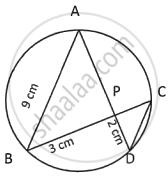
- Prove that ΔAPB ~ ΔCPD.
- Find the length of CD.
- Find area ΔAPB : area ΔCPD.
