Advertisements
Advertisements
प्रश्न
In the given figure, ∠CAB = 25°, find ∠BDC, ∠DBA and ∠COB
उत्तर
In ΔACP,
∠ACP = 180° – (25° + 90°)
= 180° – 115°
= 65°
∠CBA = ∠CAB = 25° ...[Both the angles are standing in the same base]
∠DBA = 65° ...[∠DBA and ∠BCA standing in the same base]
∠COB = 50°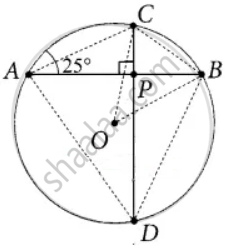
APPEARS IN
संबंधित प्रश्न
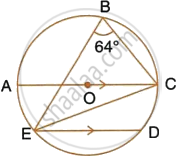
In the given figure, AOC is a diameter and AC is parallel to ED. If ∠CBE = 64°, calculate ∠DEC.
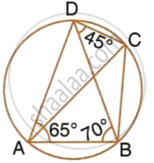
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find:
- ∠BCD
- ∠ACB
Hence, show that AC is a diameter.
In a cyclic-trapezium, the non-parallel sides are equal and the diagonals are also equal. Prove it.
In the given circle with diameter AB, find the value of x.
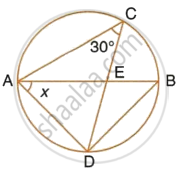
In the given figure, ∠ACE = 43° and ∠CAF = 62°; find the values of a, b and c.

In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.

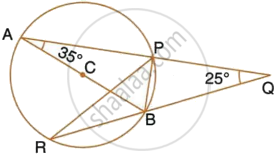
AB is a diameter of the circle APBR, as shown in the figure. APQ and RBQ are straight lines. Find : ∠BPR
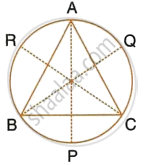
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that :
∠ACB = 2∠APR,
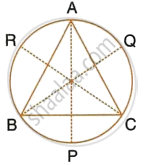
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that :
`∠QPR = 90^circ - 1/2 ∠BAC`
If I is the incentre of triangle ABC and AI when produced meets the cicrumcircle of triangle ABC in points D . if ∠BAC = 66° and ∠ABC = 80°. Calculate : ∠IBC