Advertisements
Advertisements
प्रश्न
In the given figure, CM and RN are respectively the medians of ΔABC and ΔPQR. If ΔABC ∼ ΔPQR, then prove that ΔAMC ∼ ΔPNR.

उत्तर

Given: ΔABC ∼ ΔPQR
and CM and RN are medians of ΔABC and ΔPQR respectively.
To Prove: ΔAMC ∼ ΔPNR
Proof: ΔABC ∼ ΔPQR ...(Given)
∴ ∠A = ∠P,
and `(AB)/(PQ) = (BC)/(QR) = (AC)/(PR)`
`(AB)/(PQ) = (AC)/(PR)`
`(2AM)/(2PN) = (AC)/(PR)`
`(AM)/(PN) = (AC)/(PR)`
and ∠A = ∠P
∴ ΔAMC ∼ ΔPQR ...(SAS Test)
Hence Proved.
संबंधित प्रश्न
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:

S and T are point on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ∼ ΔRTS.
Sides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Show that ΔABC ~ ΔPQR.
The sides of certain triangles are given below. Determine which of them right triangles are.
1.4cm, 4.8cm, 5cm
Two triangles ABC and PQR are such that AB = 3 cm, AC = 6cm, ∠𝐴 = 70°, PR = 9cm ∠𝑃 = 70° and PQ = 4.5 cm. Show that ΔABC ∼ΔPQR and state that similarity criterion.
In ΔABC~ΔDEF such that 2AB = DE and BC = 6cm, find EF.
In figure, if DE || BC, find the ratio of ar(ADE) and ar(DECB).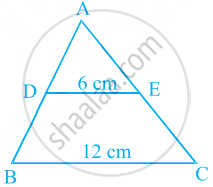
In figure, if ∠A = ∠C, AB = 6 cm, BP = 15 cm, AP = 12 cm and CP = 4 cm, then find the lengths of PD and CD.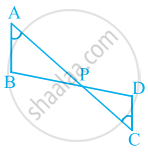
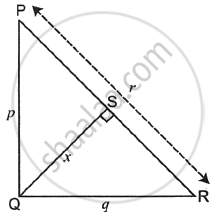
In the given figure, ΔPQR is a right-angled triangle with ∠PQR = 90°. QS is perpendicular to PR. Prove that pq = rx.
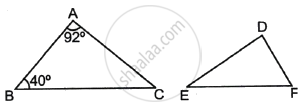
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?