Advertisements
Advertisements
प्रश्न
In figure, if ∠A = ∠C, AB = 6 cm, BP = 15 cm, AP = 12 cm and CP = 4 cm, then find the lengths of PD and CD.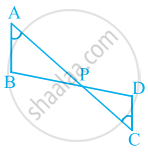
उत्तर
According to the question,
∠A = ∠C,
AB = 6 cm,
BP = 15 cm,
AP = 12 cm
CP = 4 cm
From ∆APB and ∆CPD,
∠A = ∠C
∠APB = ∠CPD ...[Vertically opposite angles]
∴ By AAA similarity criteria,
ΔAPD ∼ ΔCPD
Using basic proportionality theorem,
⇒ `("AP")/("CP") = ("PB")/("PD") = ("AB")/("CD")` ...[By basic proportionality theorem]
⇒ `12/4 = 15/("PD") = 6/("CD")`
Considering `("AP")/("CP") = ("PB")/("PD")`, we get,
`12/4 = 15/("PD")`
PD = `(15 xx 4)/12`
= `60/12`
= 5 cm
Considering, `("AP")/("CP") = ("AB")/("CD")`
⇒ CD = `((6 xx 4))/12` = 2 cm
Therefore,
Length of PD = 5 cm
Length of CD = 2 cm
APPEARS IN
संबंधित प्रश्न
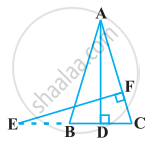
In the following figure, E is a point on side CB produced of an isosceles triangle ABC with AB = AC. If AD ⊥ BC and EF ⊥ AC, prove that ΔABD ∼ ΔECF.
The sides of certain triangles are given below. Determine which of them right triangles are.
9cm, 16cm, 18cm
In ΔABC~ΔDEF such that 2AB = DE and BC = 6cm, find EF.
In a trapezium ABCD, it is given that AB║CD and AB = 2CD. Its diagonals AC and BD intersect at the point O such that ar(ΔAOB) = 84cm2. Find ar(ΔCOD).
The corresponding sides of two similar triangles are in the ratio 2 : 3. If the area of the smaller triangle is 48 cm2, find the area of the larger triangle.
In figure, BD and CE intersect each other at the point P. Is ΔPBC ~ ΔPDE? Why?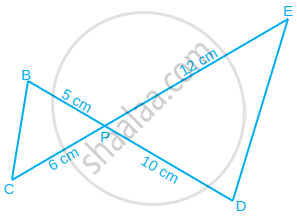
If ∆ABC ~ ∆DEF, AB = 4 cm, DE = 6 cm, EF = 9 cm and FD = 12 cm, find the perimeter of ∆ABC.
In a triangle PQR, N is a point on PR such that QN ⊥ PR. If PN . NR = QN2, prove that ∠PQR = 90°.
A 15 metres high tower casts a shadow 24 metres long at a certain time and at the same time, a telephone pole casts a shadow 16 metres long. Find the height of the telephone pole.
A tangent ADB is drawn to a circle at D whose centre is C. Also, PQ is a chord parallel to AB and ∠QDB = 50°. Find the value of ∠PDQ.

