Advertisements
Advertisements
प्रश्न
In figure, if ∠A = ∠C, AB = 6 cm, BP = 15 cm, AP = 12 cm and CP = 4 cm, then find the lengths of PD and CD.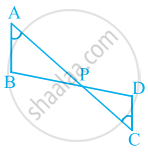
उत्तर
According to the question,
∠A = ∠C,
AB = 6 cm,
BP = 15 cm,
AP = 12 cm
CP = 4 cm
From ∆APB and ∆CPD,
∠A = ∠C
∠APB = ∠CPD ...[Vertically opposite angles]
∴ By AAA similarity criteria,
ΔAPD ∼ ΔCPD
Using basic proportionality theorem,
⇒
⇒
Considering
PD =
=
= 5 cm
Considering,
⇒ CD =
Therefore,
Length of PD = 5 cm
Length of CD = 2 cm
APPEARS IN
संबंधित प्रश्न
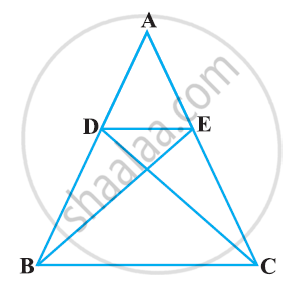
In the following figure, if ΔABE ≅ ΔACD, show that ΔADE ∼ ΔABC.
In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:
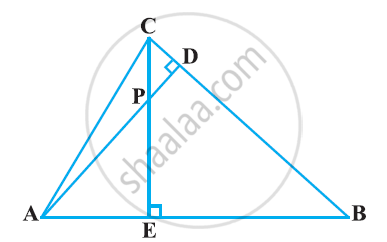
ΔAEP ∼ ΔADB
A ladder 10m long reaches the window of a house 8m above the ground. Find the distance of the foot of the ladder from the base of the wall.
In the given figure, value of x(in cm) is

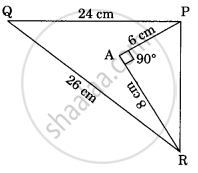
In the given figure, PQ = 24 cm, QR = 26 cm ∠PAR = 90°, PA = 6 cm, and AR = 8 cm, the degree measure of ∠QPR is ______.
In ABC, DE || AB. If CD = 3 cm, EC = 4 cm, BE = 6 cm, then DA is equal to ______.
In a triangle PQR, N is a point on PR such that QN ⊥ PR. If PN . NR = QN2, prove that ∠PQR = 90°.
If in two triangles ABC and PQR,
In the given figure, DE ∥ BC, AE = a units, EC = b units, DE = x units and BC = y units. Which of the following is true?
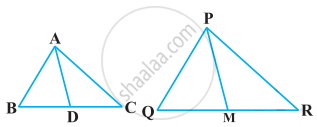
Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of ΔPQR show that ΔABC ~ ΔPQR.