Advertisements
Advertisements
प्रश्न
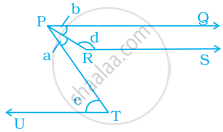
In the given figure, PQ, RS and UT are parallel lines. If c = 57° and a = `c/3`, find the value of d.
उत्तर
Since, PQ || UT and PT is transversal,
Therefore, ∠QPT = ∠UTP ......[Alternate interior angles]
⇒ a + b = c
⇒ `c/3` + b = c ......`[∵ a = c/3, "given"]`
⇒ b = `c - c/3`
⇒ b = `(3c - c)/3`
⇒ b = `(2c)/3 = 2/3 xx 57^circ` ......[∵ c = 57°, given]
∴ b = 38°
Again, PQ || RS and PR is transversal
Therefore, ∠QPR + ∠PRS = 180° ......[Consecutive interior angles]
⇒ b + d = 180°
⇒ d = 180° – b
⇒ d = 180° – 38° ......[∵ b = 38°]
⇒ d = 142°
APPEARS IN
संबंधित प्रश्न
In the figure, AB is parallel to CD, find x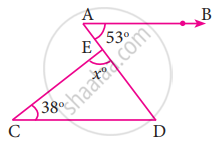
Find the measure of angle x in the following figure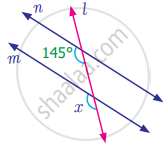
Find the measure of angle y in the following figure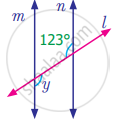
Find the measure of angle z in the following figure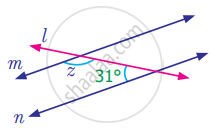
Find the measure of angle z in the following figure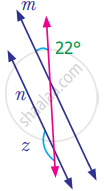
Find the value of angle a in the following figure
Which of the following statement is ALWAYS TRUE when parallel lines are cut by a transversal
Draw two parallel lines and a transversal. Mark two alternate interior angles G and H. If they are supplementary, what is the measure of each angle?
Two parallel lines are cut by a transversal. For each pair of interior angles on the same side of the transversal, if one angle exceeds the twice of the other angle by 48°. Find the angles
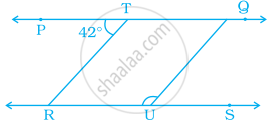
In the given figure, PQ || RS, TR || QU and ∠PTR = 42°. Find ∠QUR.