Advertisements
Advertisements
प्रश्न
In the given figure YH || TE. Prove that ΔWHY ~ ΔWET and also find HE and TE
उत्तर
| Statements | Reasons |
| 1. ∠EWT = ∠HWY | Common angle |
| 2. ∠ETW = ∠HYW | Since YH || TE, corresponding angles |
| 3. ∠WET = ∠WHY | Since YH || TE corresponding angles |
| 4. ΔWHY ~ ΔWET | By AAA criteria |
Also ΔWHY ~ ΔWET
∴ Corresponding sides are proportionated
`"WH"/"WE" = "HY"/"ET" = "WY"/"WT"`
`6/(6 + "HE") = 4/"ET" = 4/16`
`6/(6 + "HE") = 4/16`
⇒ 6 + HE = `6/4 xx 16`
⇒ 6 + HE = 24
∴ HE = 24 – 6
HE = 18
Again `4/"ET" = 4/16`
ET = `4/4 xx 16`
ET = 1 × 16
ET = 16
APPEARS IN
संबंधित प्रश्न
In the given figure, DB⊥BC, DE⊥AB and AC⊥BC.
Prove that `(BE)/(DE)=(AC)/(BC)`

In Figure 3, ABCD is a trapezium with AB || DC, AB = 18 cm, DC = 32 cm and the distance between AB and DC is 14 cm. If arcs of equal radii 7 cm have been drawn, with centres A,B, C and D, then find the area of the shaded region.

A model of a ship is made to a scale 1 : 300.
- The length of the model of the ship is 2 m. Calculate the length of the ship.
- The area of the deck of the ship is 180,000 m2. Calculate the area of the deck of the model.
- The volume of the model is 6.5 m3. Calculate the volume of the ship.
In the figure, DE || AC and DC || AP. Prove that `"BE"/"EC" = "BC"/"CP"`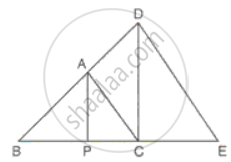
ΔABC is enlarged, with a scale factor 5. Find: A'B', if AB = 4cm
A vertical stick of length 6 m casts a shadow 400 cm long on the ground and at the same time a tower casts a shadow 28 m long. Using similarity, find the height of the tower
D is the mid point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED = x, AD = p and AE = h, prove that b2 + c2 = `2"p"^2 + "a"^2/2`
Given ΔABC ~ ΔDEF, if ∠A = 45° and ∠E = 35° then ∠B = ?
Write the test of similarity for triangles given in figure.
In the adjoining diagram the length of PR is ______.

