Advertisements
Advertisements
प्रश्न
Let \[f\left( x \right) = \frac{\alpha x}{x + 1}, x \neq - 1\] Then, for what value of α is \[f \left( f\left( x \right) \right) = x?\]
पर्याय
\[\sqrt{2}\]
\[- \sqrt{2}\]
1
-1
उत्तर
(d) −1
\[f\left( f\left( x \right) \right) = x\]
\[ \Rightarrow f\left( \frac{\alpha x}{x + 1} \right) = x\]
\[ \Rightarrow \frac{\alpha\left( \frac{\alpha x}{x + 1} \right)}{\left( \frac{\alpha x}{x + 1} \right) + 1} = x\]
\[ \Rightarrow \frac{\alpha^2 x}{\alpha x + x + 1} = x\]
\[ \Rightarrow \alpha^2 x = \alpha x^2 + x^2 + x\]
\[ \Rightarrow \alpha^2 x - \alpha x^2 - x^2 - x = 0\]
\[ \Rightarrow \alpha^2 x - \alpha x^2 - \left( x^2 + x \right) = 0\]
\[\text{Solving } \text{for } \text{ the } \alpha \text{ we get}, \]
\[\alpha = \frac{- \left( - x^2 \right) \pm \sqrt{\left( - x^2 \right)^2 - 4 \times x \times \left[ - \left( x^2 + x \right) \right]}}{2x}\]
\[ = \frac{x^2 \pm \sqrt{x^4 + 4 x^3 + 4 x^2}}{2x}\]
\[ = x + 1, - 1\]
\[\text{Here, - 1 is independent of } x, \]
\[ \therefore for, \alpha = - 1, f\left( f\left( x \right) \right) = x\]
APPEARS IN
संबंधित प्रश्न
Show that the function f in `A=R-{2/3} ` defined as `f(x)=(4x+3)/(6x-4)` is one-one and onto hence find f-1
Show that the Signum Function f: R → R, given by `f(x) = {(1, if x > 0), (0, if x = 0), (-1, if x < 0):}` is neither one-one nor onto
Let f: R → R be defined as f(x) = x4. Choose the correct answer.
Prove that the function f : N → N, defined by f(x) = x2 + x + 1, is one-one but not onto
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = sinx
Let A = [-1, 1]. Then, discuss whether the following function from A to itself is one-one, onto or bijective : g(x) = |x|
Set of ordered pair of a function ? If so, examine whether the mapping is injective or surjective :{(a, b) : a is a person, b is an ancestor of a}
If A = {1, 2, 3}, show that a one-one function f : A → A must be onto.
Find gof and fog when f : R → R and g : R → R is defined by f(x) = 8x3 and g(x) = x1/3.
Find fog (2) and gof (1) when : f : R → R ; f(x) = x2 + 8 and g : R → R; g(x) = 3x3 + 1.
Let f : R → R and g : R → R be defined by f(x) = x + 1 and g (x) = x − 1. Show that fog = gof = IR.
Find fog and gof if : f(x)= x + 1, g (x) = 2x + 3 .
Let f(x) = x2 + x + 1 and g(x) = sin x. Show that fog ≠ gof.
Let
f (x) =`{ (1 + x, 0≤ x ≤ 2) , (3 -x , 2 < x ≤ 3):}`
Find fof.
If f, g : R → R be two functions defined as f(x) = |x| + x and g(x) = |x|- x, ∀x∈R" .Then find fog and gof. Hence find fog(–3), fog(5) and gof (–2).
State with reason whether the following functions have inverse:
h : {2, 3, 4, 5} → {7, 9, 11, 13} with h = {(2, 7), (3, 9), (4, 11), (5, 13)}
Consider f : R+ → [−5, ∞) given by f(x) = 9x2 + 6x − 5. Show that f is invertible with `f^-1 (x) = (sqrt (x +6)-1)/3 .`
If f : R → (−1, 1) defined by `f (x) = (10^x- 10^-x)/(10^x + 10 ^-x)` is invertible, find f−1.
If f : A → A, g : A → A are two bijections, then prove that fog is a surjection ?
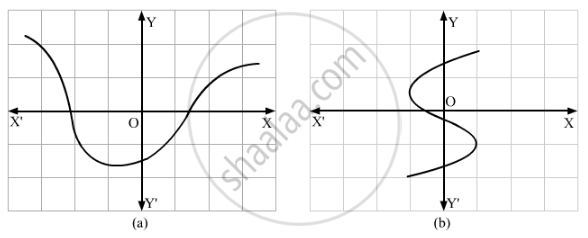
Which one of the following graphs represents a function?
If f : C → C is defined by f(x) = x2, write f−1 (−4). Here, C denotes the set of all complex numbers.
Let f : R → R be defined as `f (x) = (2x - 3)/4.` write fo f-1 (1) .
Write the domain of the real function f defined by f(x) = `sqrt (25 -x^2)` [NCERT EXEMPLAR]
If \[f\left( x \right) = \sin^2 x\] and the composite function \[g\left( f\left( x \right) \right) = \left| \sin x \right|\] then g(x) is equal to
Let \[f\left(x\right) = x^3\] be a function with domain {0, 1, 2, 3}. Then domain of \[f^{-1}\] is ______.
Mark the correct alternative in the following question:
If the set A contains 5 elements and the set B contains 6 elements, then the number of one-one and onto mappings from A to B is
Let N be the set of natural numbers and the function f: N → N be defined by f(n) = 2n + 3 ∀ n ∈ N. Then f is ______.
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
g = {(1, 4), (2, 4), (3, 4)}
Let A = R – {3}, B = R – {1}. Let f: A → B be defined by f(x) = `(x - 2)/(x - 3)` ∀ x ∈ A . Then show that f is bijective
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
f(x) = `x/2`
The function f : R → R given by f(x) = x3 – 1 is ____________.
Given a function If as f(x) = 5x + 4, x ∈ R. If g : R → R is inverse of function ‘f then
Let f(1, 3) `rightarrow` R be a function defined by f(x) = `(x[x])/(1 + x^2)`, where [x] denotes the greatest integer ≤ x, Then the range of f is ______.
Let f(n) = `[1/3 + (3n)/100]n`, where [n] denotes the greatest integer less than or equal to n. Then `sum_(n = 1)^56f(n)` is equal to ______.
If A = {x ∈ R: |x – 2| > 1}, B = `{x ∈ R : sqrt(x^2 - 3) > 1}`, C = {x ∈ R : |x – 4| ≥ 2} and Z is the set of all integers, then the number of subsets of the set (A ∩ B ∩ C) C ∩ Z is ______.
Let a function `f: N rightarrow N` be defined by
f(n) = `{:[(2n",", n = 2"," 4"," 6"," 8","......),(n - 1",", n = 3"," 7"," 11"," 15","......),((n + 1)/2",", n = 1"," 5"," 9"," 13","......):}`
then f is ______.
The function f(x) = [x], where [x] denotes the greatest integer less than or equal to x; is continuous at ______.
Let f(x) be a polynomial function of degree 6 such that `d/dx (f(x))` = (x – 1)3 (x – 3)2, then
Assertion (A): f(x) has a minimum at x = 1.
Reason (R): When `d/dx (f(x)) < 0, ∀ x ∈ (a - h, a)` and `d/dx (f(x)) > 0, ∀ x ∈ (a, a + h)`; where 'h' is an infinitesimally small positive quantity, then f(x) has a minimum at x = a, provided f(x) is continuous at x = a.
The trigonometric equation tan–1x = 3tan–1 a has solution for ______.
