Advertisements
Advertisements
प्रश्न
Let A = R – {3}, B = R – {1}. Let f: A → B be defined by f(x) = `(x - 2)/(x - 3)` ∀ x ∈ A . Then show that f is bijective
उत्तर
Given that,
A = R – {3}, B = R – {1}
f: A → B be defined by f(x) = `(x - 2)/x` – 3 ∀ x ∈ A
∴ f(x) = `(x - 3 + 1)/(x - 3)` = 1 + `1/(x - 3)`
Let f(x1) = f (x2)
⇒ `1 + 1/(x_1 - 3) = 1 + 1/(x_2 - 3)`
⇒ `1/(x_1 - 3) = 1/(x_2 - 3)`
⇒ x1 = x2
So, (x) is an injection function
Now let y = `(x - 2)/(x - 3)`
⇒ x – 2 = xy – 3y
⇒ x(1 – y) = 2 – 3y
⇒ x = `(2 - 3y)/(1 - y)`
⇒ x = `(3y - 2)/(y - 1)`
⇒ y ∈ R – {1} = B
इसलिए f(x) आच्छादक या व्यक्तिपरक है।
अत: f(x) एक विशेषण फलन है।
APPEARS IN
संबंधित प्रश्न
Show that the function f: R* → R* defined by `f(x) = 1/x` is one-one and onto, where R* is the set of all non-zero real numbers. Is the result true if the domain R* is replaced by N, with co-domain being same as R?
Show that the modulus function f: R → R given by f(x) = |x| is neither one-one nor onto, where |x| is x, if x is positive or 0 and |x| is − x if x is negative.
Let f: R → R be the Signum Function defined as
f(x) = `{(1,x>0), (0, x =0),(-1, x< 0):}`
and g: R → R be the Greatest Integer Function given by g(x) = [x], where [x] is greatest integer less than or equal to x. Then does fog and gof coincide in (0, 1]?
Give examples of two surjective functions f1 and f2 from Z to Z such that f1 + f2 is not surjective.
Give examples of two functions f : N → Z and g : Z → Z, such that gof is injective but gis not injective.
Find fog and gof if : f (x) = x+1, g(x) = `e^x`
.
Find fog and gof if : f(x)= x + 1, g (x) = 2x + 3 .
Let f : R `{- 4/3} `- 43 →">→ R be a function defined as f(x) = `(4x)/(3x +4)` . Show that f : R - `{-4/3}`→ Rang (f) is one-one and onto. Hence, find f -1.
If f : R → (−1, 1) defined by `f (x) = (10^x- 10^-x)/(10^x + 10 ^-x)` is invertible, find f−1.
Let f : R − {−1} → R − {1} be given by\[f\left( x \right) = \frac{x}{x + 1} . \text{Write } f^{- 1} \left( x \right)\]
What is the range of the function
`f (x) = ([x - 1])/(x -1) ?`
If f : R → R be defined by f(x) = (3 − x3)1/3, then find fof (x).
Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. State whether f is one-one or not.
Let\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = \text{B and C} = \left\{ x \in R : x \geq 0 \right\} and\]\[S = \left\{ \left( x, y \right) \in A \times B : x^2 + y^2 = 1 \right\} \text{and } S_0 = \left\{ \left( x, y \right) \in A \times C : x^2 + y^2 = 1 \right\}\]
Then,
Let
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = B\] Then, the mapping\[f : A \to \text{B given by} f\left( x \right) = x\left| x \right|\] is
Let M be the set of all 2 × 2 matrices with entries from the set R of real numbers. Then, the function f : M→ R defined by f(A) = |A| for every A ∈ M, is
Let
\[f : R \to R\] be a function defined by
The distinct linear functions that map [−1, 1] onto [0, 2] are
Mark the correct alternative in the following question:
Let f : R → R be given by f(x) = tanx. Then, f-1(1) is
Which function is used to check whether a character is alphanumeric or not?
Let R be the set of real numbers and f: R → R be the function defined by f(x) = 4x + 5. Show that f is invertible and find f–1.
Let f: R → R be defined by f(x) = 3x – 4. Then f–1(x) is given by ______.
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
f = {(1, 4), (1, 5), (2, 4), (3, 5)}
Let A = {1, 2, 3, ...n} and B = {a, b}. Then the number of surjections from A into B is ______.
Let f: R – `{3/5}` → R be defined by f(x) = `(3x + 2)/(5x - 3)`. Then ______.
Let f: `[2, oo)` → R be the function defined by f(x) = x2 – 4x + 5, then the range of f is ______.
Let A = {0, 1} and N be the set of natural numbers. Then the mapping f: N → A defined by f(2n – 1) = 0, f(2n) = 1, ∀ n ∈ N, is onto.
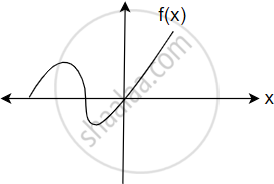
The given function f : R → R is not ‘onto’ function. Give reason.
