Advertisements
Advertisements
प्रश्न
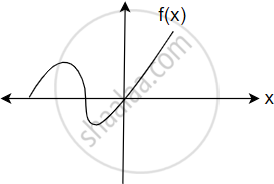
The given function f : R → R is not ‘onto’ function. Give reason.
उत्तर
Since each line in co-domain of the function parallel to x-axis, doesn’t cuts the graph of function at least one point, therefore the f(x) is not an onto function.
APPEARS IN
संबंधित प्रश्न
Show that the modulus function f: R → R given by f(x) = |x| is neither one-one nor onto, where |x| is x, if x is positive or 0 and |x| is − x if x is negative.
Let f: R → R be defined as f(x) = 3x. Choose the correct answer.
Which of the following functions from A to B are one-one and onto?
f1 = {(1, 3), (2, 5), (3, 7)} ; A = {1, 2, 3}, B = {3, 5, 7}
Prove that the function f : N → N, defined by f(x) = x2 + x + 1, is one-one but not onto
Classify the following function as injection, surjection or bijection : f : Z → Z given by f(x) = x3
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = sin2x + cos2x
Set of ordered pair of a function ? If so, examine whether the mapping is injective or surjective :{(a, b) : a is a person, b is an ancestor of a}
Let A = {1, 2, 3}. Write all one-one from A to itself.
Give examples of two one-one functions f1 and f2 from R to R, such that f1 + f2 : R → R. defined by (f1 + f2) (x) = f1 (x) + f2 (x) is not one-one.
Let f(x) = x2 + x + 1 and g(x) = sin x. Show that fog ≠ gof.
Let A = R - {3} and B = R - {1}. Consider the function f : A → B defined by f(x) = `(x-2)/(x-3).`Show that f is one-one and onto and hence find f-1.
[CBSE 2012, 2014]
Which of the following graphs represents a one-one function?

Write whether f : R → R, given by `f(x) = x + sqrtx^2` is one-one, many-one, onto or into.
Write the domain of the real function f defined by f(x) = `sqrt (25 -x^2)` [NCERT EXEMPLAR]
Let\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = \text{B and C} = \left\{ x \in R : x \geq 0 \right\} and\]\[S = \left\{ \left( x, y \right) \in A \times B : x^2 + y^2 = 1 \right\} \text{and } S_0 = \left\{ \left( x, y \right) \in A \times C : x^2 + y^2 = 1 \right\}\]
Then,
Let
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = B\] Then, the mapping\[f : A \to \text{B given by} f\left( x \right) = x\left| x \right|\] is
Let
\[A = \left\{ x \in R : x \geq 1 \right\}\] The inverse of the function,
\[f : A \to A\] given by
\[f\left( x \right) = 2^{x \left( x - 1 \right)} , is\]
If \[f : R \to \left( - 1, 1 \right)\] is defined by
\[f\left( x \right) = \frac{- x|x|}{1 + x^2}, \text{ then } f^{- 1} \left( x \right)\] equals
If \[f : R \to R\] is given by \[f\left( x \right) = x^3 + 3, \text{then} f^{- 1} \left( x \right)\] is equal to
If f(x) = `(x+3)/(4x−5) , "g"(x) = (3+5x)/(4x−1)` then verify that `("fog") (x)` = x.
Which function is used to check whether a character is alphanumeric or not?
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(x, y): x is a person, y is the mother of x}
Using the definition, prove that the function f: A→ B is invertible if and only if f is both one-one and onto
Let f: R – `{3/5}` → R be defined by f(x) = `(3x + 2)/(5x - 3)`. Then ______.
Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. Based on the given information, f is best defined as:
Sherlin and Danju are playing Ludo at home during Covid-19. While rolling the dice, Sherlin’s sister Raji observed and noted the possible outcomes of the throw every time belongs to set {1,2,3,4,5,6}. Let A be the set of players while B be the set of all possible outcomes.
A = {S, D}, B = {1,2,3,4,5,6}
- Raji wants to know the number of functions from A to B. How many number of functions are possible?
Let f: R → R defined by f(x) = 3x. Choose the correct answer
If `f : R -> R^+ U {0}` be defined by `f(x) = x^2, x ∈ R`. The mapping is
The solution set of the inequation log1/3(x2 + x + 1) + 1 > 0 is ______.
The domain of function is f(x) = `sqrt(-log_0.3(x - 1))/sqrt(x^2 + 2x + 8)` is ______.
