Advertisements
Advertisements
प्रश्न
Let
\[A = \left\{ x \in R : x \geq 1 \right\}\] The inverse of the function,
\[f : A \to A\] given by
\[f\left( x \right) = 2^{x \left( x - 1 \right)} , is\]
पर्याय
\[\left( \frac{1}{2} \right)^{x \left( x - 1 \right)}\]
\[\frac{1}{2} \left\{ 1 + \sqrt{1 + 4 \log_2 x} \right\}\]
\[\frac{1}{2} \left\{ 1 - \sqrt{1 + 4 \log_2 x} \right\}\]
not defined
उत्तर
\[\text{Let} f^{- 1} \left( x \right) = y . . . \left( 1 \right)\]
\[ \Rightarrow f\left( y \right) = x\]
\[ \Rightarrow 2^{y\left( y - 1 \right)} = x\]
\[ \Rightarrow 2^{y^2 - y} = x\]
\[ \Rightarrow y^2 - y = \log_2 x\]
\[ \Rightarrow y^2 - y + \frac{1}{4} = \log_2 x + \frac{1}{4}\]
\[ \Rightarrow \left( y - \frac{1}{2} \right)^2 = \frac{4 \log_2 x + 1}{4}\]
\[ \Rightarrow y - \frac{1}{2} = \pm \frac{\sqrt{4 \log_2 x + 1}}{2}\]
\[ \Rightarrow y = \frac{1}{2} \pm \frac{\sqrt{4 \log_2 x + 1}}{2}\]
\[ \Rightarrow y = \frac{1}{2} + \frac{\sqrt{4 \log_2 x + 1}}{2} \left( \because y \geq1 \right)\]
\[So, f^{- 1} \left( x \right) = \frac{1}{2}(1 + \sqrt{1 + 4 \log_2 x} ) [\text{from}\left( 1 \right)]\]
So, the answer is (b).
APPEARS IN
संबंधित प्रश्न
Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. Show that f is one-one.
Let f: R → R be defined as f(x) = x4. Choose the correct answer.
Let S = {a, b, c} and T = {1, 2, 3}. Find F−1 of the following functions F from S to T, if it exists.
F = {(a, 3), (b, 2), (c, 1)}
Let S = {a, b, c} and T = {1, 2, 3}. Find F−1 of the following functions F from S to T, if it exists.
F = {(a, 2), (b, 1), (c, 1)}
Let f: R → R be the Signum Function defined as
f(x) = `{(1,x>0), (0, x =0),(-1, x< 0):}`
and g: R → R be the Greatest Integer Function given by g(x) = [x], where [x] is greatest integer less than or equal to x. Then does fog and gof coincide in (0, 1]?
Show that the function f: ℝ → ℝ defined by f(x) = `x/(x^2 + 1), ∀x in R`is neither one-one nor onto. Also, if g: ℝ → ℝ is defined as g(x) = 2x - 1. Find fog(x)
Give an example of a function which is neither one-one nor onto ?
Set of ordered pair of a function? If so, examine whether the mapping is injective or surjective :{(x, y) : x is a person, y is the mother of x}
Let A = {1, 2, 3}. Write all one-one from A to itself.
Show that if f1 and f2 are one-one maps from R to R, then the product f1 × f2 : R → R defined by (f1 × f2) (x) = f1 (x) f2 (x) need not be one - one.
If f : A → B and g : B → C are one-one functions, show that gof is a one-one function.
Let f be any real function and let g be a function given by g(x) = 2x. Prove that gof = f + f.
Find f −1 if it exists : f : A → B, where A = {1, 3, 5, 7, 9}; B = {0, 1, 9, 25, 49, 81} and f(x) = x2
Consider f : {1, 2, 3} → {a, b, c} and g : {a, b, c} → {apple, ball, cat} defined as f (1) = a, f (2) = b, f (3) = c, g (a) = apple, g (b) = ball and g (c) = cat. Show that f, g and gof are invertible. Find f−1, g−1 and gof−1and show that (gof)−1 = f −1o g−1
Show that the function f : Q → Q, defined by f(x) = 3x + 5, is invertible. Also, find f−1
If f : R → R is defined by f(x) = 10 x − 7, then write f−1 (x).
If f : R → R be defined by f(x) = (3 − x3)1/3, then find fof (x).
Let\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = \text{B and C} = \left\{ x \in R : x \geq 0 \right\} and\]\[S = \left\{ \left( x, y \right) \in A \times B : x^2 + y^2 = 1 \right\} \text{and } S_0 = \left\{ \left( x, y \right) \in A \times C : x^2 + y^2 = 1 \right\}\]
Then,
\[f : R \to R \text{given by} f\left( x \right) = x + \sqrt{x^2} \text{ is }\]
Let
f : R → R be given by
\[f\left( x \right) = \left[ x^2 \right] + \left[ x + 1 \right] - 3\]
where [x] denotes the greatest integer less than or equal to x. Then, f(x) is
(d) one-one and onto
Let f be an injective map with domain {x, y, z} and range {1, 2, 3}, such that exactly one of the following statements is correct and the remaining are false.
\[f\left( x \right) = 1, f\left( y \right) \neq 1, f\left( z \right) \neq 2 .\]
The value of
\[f^{- 1} \left( 1 \right)\] is
Which function is used to check whether a character is alphanumeric or not?
Let f: R → R be defined by f(x) = x2 + 1. Then, pre-images of 17 and – 3, respectively, are ______.
Which of the following functions from Z into Z are bijections?
The function f : A → B defined by f(x) = 4x + 7, x ∈ R is ____________.
The function f : R → R defined by f(x) = 3 – 4x is ____________.
Which of the following functions from Z into Z is bijective?
Let f : R `->` R be a function defined by f(x) = x3 + 4, then f is ______.
The domain of the function `"f"("x") = 1/(sqrt ({"sin x"} + {"sin" ( pi + "x")}))` where {.} denotes fractional part, is
A general election of Lok Sabha is a gigantic exercise. About 911 million people were eligible to vote and voter turnout was about 67%, the highest ever

Let I be the set of all citizens of India who were eligible to exercise their voting right in the general election held in 2019. A relation ‘R’ is defined on I as follows:
R = {(V1, V2) ∶ V1, V2 ∈ I and both use their voting right in the general election - 2019}
- Mr. ’X’ and his wife ‘W’ both exercised their voting right in the general election-2019, Which of the following is true?
An organization conducted a bike race under 2 different categories-boys and girls. Totally there were 250 participants. Among all of them finally, three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project. Let B = {b1,b2,b3} G={g1,g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.
Ravi decides to explore these sets for various types of relations and functions.
- Let R: B → G be defined by R = { (b1,g1), (b2,g2),(b3,g1)}, then R is ____________.
An organization conducted a bike race under 2 different categories-boys and girls. Totally there were 250 participants. Among all of them finally, three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project. Let B = {b1,b2,b3} G={g1,g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.
Ravi decides to explore these sets for various types of relations and functions.
- Ravi wants to find the number of injective functions from B to G. How many numbers of injective functions are possible?
Let x is a real number such that are functions involved are well defined then the value of `lim_(t→0)[max{(sin^-1 x/3 + cos^-1 x/3)^2, min(x^2 + 4x + 7)}]((sin^-1t)/t)` where [.] is greatest integer function and all other brackets are usual brackets.
If f: [0, 1]→[0, 1] is defined by f(x) = `(x + 1)/4` and `d/(dx) underbrace(((fofof......of)(x)))_("n" "times")""|_(x = 1/2) = 1/"m"^"n"`, m ∈ N, then the value of 'm' is ______.
Let f: R→R be a polynomial function satisfying f(x + y) = f(x) + f(y) + 3xy(x + y) –1 ∀ x, y ∈ R and f'(0) = 1, then `lim_(x→∞)(f(2x))/(f(x)` is equal to ______.
Let A = {1, 2, 3, ..., 10} and f : A `rightarrow` A be defined as
f(k) = `{{:(k + 1, if k "is odd"),( k, if k "is even"):}`.
Then the number of possible functions g : A `rightarrow` A such that gof = f is ______.
A function f : [– 4, 4] `rightarrow` [0, 4] is given by f(x) = `sqrt(16 - x^2)`. Show that f is an onto function but not a one-one function. Further, find all possible values of 'a' for which f(a) = `sqrt(7)`.
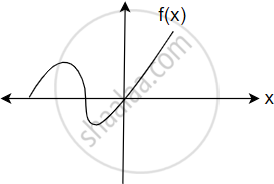
The given function f : R → R is not ‘onto’ function. Give reason.
