Advertisements
Advertisements
प्रश्न
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(x, y): x is a person, y is the mother of x}
उत्तर
Given, {(x, y): x is a person, y is the mother of x}
It’s clearly seen that each person ‘x’ has only one biological mother.
Hence, the above set of ordered pairs make a function.
Now more than one person may have same mother.
Thus, the function is many-many one and surjective.
APPEARS IN
संबंधित प्रश्न
Check the injectivity and surjectivity of the following function:
f: N → N given by f(x) = x2
Check the injectivity and surjectivity of the following function:
f: R → R given by f(x) = x2
Check the injectivity and surjectivity of the following function:
f: Z → Z given by f(x) = x3
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 3 − 4x
If f : A → B is an injection, such that range of f = {a}, determine the number of elements in A.
Show that the function f : R − {3} → R − {2} given by f(x) = `(x-2)/(x-3)` is a bijection.
Let A = [-1, 1]. Then, discuss whether the following functions from A to itself is one-one, onto or bijective : h(x) = x2
Show that the logarithmic function f : R0+ → R given by f (x) loga x ,a> 0 is a bijection.
Give examples of two functions f : N → N and g : N → N, such that gof is onto but f is not onto.
Find fog and gof if : f(x) = sin−1 x, g(x) = x2
Let f be a function from R to R, such that f(x) = cos (x + 2). Is f invertible? Justify your answer.
If f : A → A, g : A → A are two bijections, then prove that fog is an injection ?
If A = {1, 2, 3} and B = {a, b}, write the total number of functions from A to B.
Let f : R − {−1} → R − {1} be given by\[f\left( x \right) = \frac{x}{x + 1} . \text{Write } f^{- 1} \left( x \right)\]
Let f : R → R, g : R → R be two functions defined by f(x) = x2 + x + 1 and g(x) = 1 − x2. Write fog (−2).
If f(x) = x + 7 and g(x) = x − 7, x ∈ R, write fog (7).
\[f : A \to \text{B given by } 3^{ f\left( x \right)} + 2^{- x} = 4\] is a bijection, then
The function \[f : [0, \infty ) \to \text {R given by } f\left( x \right) = \frac{x}{x + 1} is\]
If A = {a, b, c, d} and f = {a, b), (b, d), (c, a), (d, c)}, show that f is one-one from A onto A. Find f–1
Consider the set A containing n elements. Then, the total number of injective functions from A onto itself is ______
The function f : A → B defined by f(x) = 4x + 7, x ∈ R is ____________.
Let f : [0, ∞) → [0, 2] be defined by `"f" ("x") = (2"x")/(1 + "x"),` then f is ____________.
If N be the set of all-natural numbers, consider f: N → N such that f(x) = 2x, ∀ x ∈ N, then f is ____________.
An organization conducted a bike race under 2 different categories-boys and girls. Totally there were 250 participants. Among all of them finally, three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project. Let B = {b1,b2,b3} G={g1,g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.
Ravi decides to explore these sets for various types of relations and functions.
- Ravi wants to know among those relations, how many functions can be formed from B to G?
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.
Answer the following questions using the above information.
- Let f: N → N be defined by f(x) = x2 is ____________.
Let n(A) = 4 and n(B) = 6, Then the number of one – one functions from 'A' to 'B' is:
Consider a function f: `[0, pi/2] ->` R, given by f(x) = sinx and `g[0, pi/2] ->` R given by g(x) = cosx then f and g are
The domain of the function `cos^-1((2sin^-1(1/(4x^2-1)))/π)` is ______.
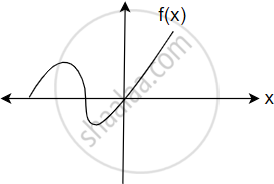
The given function f : R → R is not ‘onto’ function. Give reason.
