Advertisements
Advertisements
Question
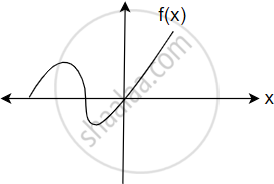
The given function f : R → R is not ‘onto’ function. Give reason.
Solution
Since each line in co-domain of the function parallel to x-axis, doesn’t cuts the graph of function at least one point, therefore the f(x) is not an onto function.
APPEARS IN
RELATED QUESTIONS
Show that the function f: R → R given by f(x) = x3 is injective.
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
If A = {1, 2, 3}, show that a one-one function f : A → A must be onto.
Give examples of two surjective functions f1 and f2 from Z to Z such that f1 + f2 is not surjective.
Suppose f1 and f2 are non-zero one-one functions from R to R. Is `f_1 / f^2` necessarily one - one? Justify your answer. Here,`f_1/f_2 : R → R is given by (f_1/f_2) (x) = (f_1(x))/(f_2 (x)) for all x in R .`
Find fog and gof if : f(x)= x + 1, g (x) = 2x + 3 .
Find fog and gof if : f(x) = `x^2` + 2 , g (x) = 1 − `1/ (1-x)`.
Let
f (x) =`{ (1 + x, 0≤ x ≤ 2) , (3 -x , 2 < x ≤ 3):}`
Find fof.
State with reason whether the following functions have inverse:
h : {2, 3, 4, 5} → {7, 9, 11, 13} with h = {(2, 7), (3, 9), (4, 11), (5, 13)}
Find f −1 if it exists : f : A → B, where A = {0, −1, −3, 2}; B = {−9, −3, 0, 6} and f(x) = 3 x.
Show that the function f : Q → Q, defined by f(x) = 3x + 5, is invertible. Also, find f−1
If f : R → (−1, 1) defined by `f (x) = (10^x- 10^-x)/(10^x + 10 ^-x)` is invertible, find f−1.
If A = {1, 2, 3} and B = {a, b}, write the total number of functions from A to B.
If f : R → R is given by f(x) = x3, write f−1 (1).
If f : R → R, g : R → are given by f(x) = (x + 1)2 and g(x) = x2 + 1, then write the value of fog (−3).
If f : {5, 6} → {2, 3} and g : {2, 3} → {5, 6} are given by f = {(5, 2), (6, 3)} and g = {(2, 5), (3, 6)}, then find fog. [NCERT EXEMPLAR]
The function
f : A → B defined by
f (x) = - x2 + 6x - 8 is a bijection if
The range of the function
\[f\left( x \right) =^{7 - x} P_{x - 3}\]
Let
\[f : R \to R\] be a function defined by
The function \[f : R \to R\] defined by
\[f\left( x \right) = 6^x + 6^{|x|}\] is
Mark the correct alternative in the following question:
Let f : R → R be given by f(x) = tanx. Then, f-1(1) is
Write about strlen() function.
Let the function f: R → R be defined by f(x) = 4x – 1, ∀ x ∈ R. Then, show that f is one-one.
Let f, g: R → R be two functions defined as f(x) = |x| + x and g(x) = x – x ∀ x ∈ R. Then, find f o g and g o f
If the set A contains 5 elements and the set B contains 6 elements, then the number of one-one and onto mappings from A to B is ______.
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.
Answer the following questions using the above information.
- Let f: N → N be defined by f(x) = x2 is ____________.
If f: R→R is a function defined by f(x) = `[x - 1]cos((2x - 1)/2)π`, where [ ] denotes the greatest integer function, then f is ______.
`x^(log_5x) > 5` implies ______.
Let f: R→R be a polynomial function satisfying f(x + y) = f(x) + f(y) + 3xy(x + y) –1 ∀ x, y ∈ R and f'(0) = 1, then `lim_(x→∞)(f(2x))/(f(x)` is equal to ______.
Which one of the following graphs is a function of x?
 |
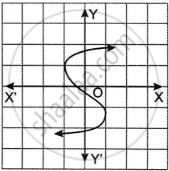 |
| Graph A | Graph B |
