Advertisements
Advertisements
Question
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
Solution
f : R → R, defined by f(x) = x3 − x
Injection test :
Let x and y be any two elements in the domain (R), such that f(x) = f(y).
f(x) = f(y)
x3−x=y3−y
Here, we cannot say x=y.
For example, x=1 and y=-1
x3−x =1−1= 0
y3−y=(−1)3−(−1)−1+1=0
So, 1 and -1 have the same image 0 .
So, f is not an injection.
Surjection test :
Let y be any element in the co-domain (R), such that f(x) = y for some element x in R (domain).
f(x) = y
x3 − x = y
By observation we can say that there exist some x in R, such that x3 - x = y.
So, f is a surjection and f is not a bijection.
APPEARS IN
RELATED QUESTIONS
Check the injectivity and surjectivity of the following function:
f: N → N given by f(x) = x2
Check the injectivity and surjectivity of the following function:
f: Z → Z given by f(x) = x2
Show that the function f: R → R given by f(x) = x3 is injective.
Let A = {−1, 0, 1, 2}, B = {−4, −2, 0, 2} and f, g: A → B be functions defined by f(x) = x2 − x, x ∈ A and g(x) = `2|x - 1/2|- 1, x in A`. Are f and g equal?
Justify your answer. (Hint: One may note that two functions f: A → B and g: A → B such that f(a) = g(a) ∀ a ∈ A are called equal functions).
Classify the following function as injection, surjection or bijection :
f : Z → Z, defined by f(x) = x2 + x
Classify the following function as injection, surjection or bijection :
f : Q − {3} → Q, defined by `f (x) = (2x +3)/(x-3)`
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 3 − 4x
Let A = {1, 2, 3}. Write all one-one from A to itself.
Let f = {(1, −1), (4, −2), (9, −3), (16, 4)} and g = {(−1, −2), (−2, −4), (−3, −6), (4, 8)}. Show that gof is defined while fog is not defined. Also, find gof.
Give examples of two functions f : N → Z and g : Z → Z, such that gof is injective but gis not injective.
Let A = {1, 2, 3, 4}; B = {3, 5, 7, 9}; C = {7, 23, 47, 79} and f : A → B, g : B → C be defined as f(x) = 2x + 1 and g(x) = x2 − 2. Express (gof)−1 and f−1 og−1 as the sets of ordered pairs and verify that (gof)−1 = f−1 og−1.
If f : A → A, g : A → A are two bijections, then prove that fog is an injection ?
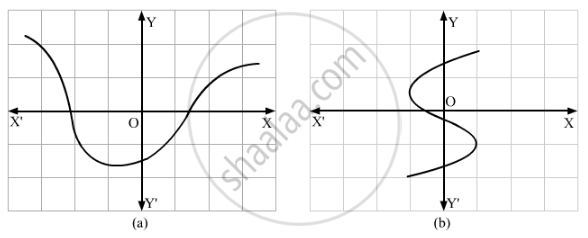
Which one of the following graphs represents a function?
Let C denote the set of all complex numbers. A function f : C → C is defined by f(x) = x3. Write f−1(1).
Let A = {x ∈ R : −4 ≤ x ≤ 4 and x ≠ 0} and f : A → R be defined by \[f\left( x \right) = \frac{\left| x \right|}{x}\]Write the range of f.
Let \[f : \left[ - \frac{\pi}{2}, \frac{\pi}{2} \right] \to\] A be defined by f(x) = sin x. If f is a bijection, write set A.
Write the domain of the real function
`f (x) = sqrt([x] - x) .`
What is the range of the function
`f (x) = ([x - 1])/(x -1) ?`
Let f : R → R be the function defined by f(x) = 4x − 3 for all x ∈ R Then write f . [NCERT EXEMPLAR]
Let M be the set of all 2 × 2 matrices with entries from the set R of real numbers. Then, the function f : M→ R defined by f(A) = |A| for every A ∈ M, is
The function f : [-1/2, 1/2, 1/2] → [-π /2,π/2], defined by f (x) = `sin^-1` (3x - `4x^3`), is
Which of the following functions from
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\}\]
Let
\[f : [2, \infty ) \to X\] be defined by
\[f\left( x \right) = 4x - x^2\] Then, f is invertible if X =
Let \[f\left(x\right) = x^3\] be a function with domain {0, 1, 2, 3}. Then domain of \[f^{-1}\] is ______.
Let A = R − (2) and B = R − (1). If f: A ⟶ B is a function defined by`"f(x)"=("x"-1)/("x"-2),` how that f is one-one and onto. Hence, find f−1.
Let f, g: R → R be two functions defined as f(x) = |x| + x and g(x) = x – x ∀ x ∈ R. Then, find f o g and g o f
Let A be a finite set. Then, each injective function from A into itself is not surjective.
For sets A, B and C, let f: A → B, g: B → C be functions such that g o f is surjective. Then g is surjective.
Let the function f: R → R be defined by f(x) = cosx, ∀ x ∈ R. Show that f is neither one-one nor onto
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
f(x) = `x/2`
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
g(x) = |x|
If f(x) = (4 – (x – 7)3}, then f–1(x) = ______.
Let X = {-1, 0, 1}, Y = {0, 2} and a function f : X → Y defiend by y = 2x4, is ____________.
If `f : R -> R^+ U {0}` be defined by `f(x) = x^2, x ∈ R`. The mapping is
A function f: x → y is said to be one – one (or injective) if:
Consider a function f: `[0, pi/2] ->` R, given by f(x) = sinx and `g[0, pi/2] ->` R given by g(x) = cosx then f and g are
Let f: R→R be a continuous function such that f(x) + f(x + 1) = 2, for all x ∈ R. If I1 = `int_0^8f(x)dx` and I2 = `int_(-1)^3f(x)dx`, then the value of I1 + 2I2 is equal to ______.
If A = {x ∈ R: |x – 2| > 1}, B = `{x ∈ R : sqrt(x^2 - 3) > 1}`, C = {x ∈ R : |x – 4| ≥ 2} and Z is the set of all integers, then the number of subsets of the set (A ∩ B ∩ C) C ∩ Z is ______.
Let f(x) be a polynomial of degree 3 such that f(k) = `-2/k` for k = 2, 3, 4, 5. Then the value of 52 – 10f(10) is equal to ______.
The trigonometric equation tan–1x = 3tan–1 a has solution for ______.
