Advertisements
Advertisements
Question
If f(x) = (4 – (x – 7)3}, then f–1(x) = ______.
Solution
If f(x) = (4 – (x – 7)3}, then f–1(x) = `7 + (4 - x)^(1/3)`.
Explanation:
Given that, f(x) = [4 – (x – 7)3]
Let y = [4 – (x – 7)3]
⇒ (x – 7)3) = 4 – y
⇒ (x – 7) = `(4 - y)^(1/3)`
⇒ x = 7 + `(4 - y)^(1/3)`
⇒ f–1(x) = `7 + (4 - x)^(1/3)`
APPEARS IN
RELATED QUESTIONS
Check the injectivity and surjectivity of the following function:
f: Z → Z given by f(x) = x3
Show that the Signum Function f: R → R, given by `f(x) = {(1, if x > 0), (0, if x = 0), (-1, if x < 0):}` is neither one-one nor onto
If the function `f(x) = sqrt(2x - 3)` is invertible then find its inverse. Hence prove that `(fof^(-1))(x) = x`
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
Let A = [-1, 1]. Then, discuss whether the following function from A to itself is one-one, onto or bijective : g(x) = |x|
Show that the exponential function f : R → R, given by f(x) = ex, is one-one but not onto. What happens if the co-domain is replaced by`R0^+` (set of all positive real numbers)?
Give examples of two one-one functions f1 and f2 from R to R, such that f1 + f2 : R → R. defined by (f1 + f2) (x) = f1 (x) + f2 (x) is not one-one.
Suppose f1 and f2 are non-zero one-one functions from R to R. Is `f_1 / f^2` necessarily one - one? Justify your answer. Here,`f_1/f_2 : R → R is given by (f_1/f_2) (x) = (f_1(x))/(f_2 (x)) for all x in R .`
If f(x) = |x|, prove that fof = f.
Consider f : R+ → [−5, ∞) given by f(x) = 9x2 + 6x − 5. Show that f is invertible with `f^-1 (x) = (sqrt (x +6)-1)/3 .`
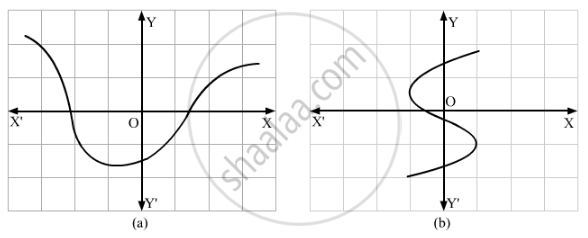
Which one of the following graphs represents a function?
Write the domain of the real function f defined by f(x) = `sqrt (25 -x^2)` [NCERT EXEMPLAR]
The range of the function
\[f\left( x \right) =^{7 - x} P_{x - 3}\]
Let
\[f : R \to R\] be a function defined by
Let
\[f : R - \left\{ n \right\} \to R\]
Which of the following functions from
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\}\]
If \[f\left( x \right) = \sin^2 x\] and the composite function \[g\left( f\left( x \right) \right) = \left| \sin x \right|\] then g(x) is equal to
Let \[f\left(x\right) = x^3\] be a function with domain {0, 1, 2, 3}. Then domain of \[f^{-1}\] is ______.
Mark the correct alternative in the following question:
If the set A contains 5 elements and the set B contains 6 elements, then the number of one-one and onto mappings from A to B is
Set A has 3 elements and the set B has 4 elements. Then the number of injective mappings that can be defined from A to B is ______.
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
k = {(1,4), (2, 5)}
If N be the set of all-natural numbers, consider f: N → N such that f(x) = 2x, ∀ x ∈ N, then f is ____________.
Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. Based on the given information, f is best defined as:
Sherlin and Danju are playing Ludo at home during Covid-19. While rolling the dice, Sherlin’s sister Raji observed and noted the possible outcomes of the throw every time belongs to set {1,2,3,4,5,6}. Let A be the set of players while B be the set of all possible outcomes.
A = {S, D}, B = {1,2,3,4,5,6}
- Raji wants to know the number of functions from A to B. How many number of functions are possible?
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.
Answer the following questions using the above information.
- Let f: R → R be defined by f(x) = x2 is:
Let the function f: R → R be defined by f(x) = 4x – 1, ∀ x ∈ R then 'f' is
Let x is a real number such that are functions involved are well defined then the value of `lim_(t→0)[max{(sin^-1 x/3 + cos^-1 x/3)^2, min(x^2 + 4x + 7)}]((sin^-1t)/t)` where [.] is greatest integer function and all other brackets are usual brackets.
Let f(n) = `[1/3 + (3n)/100]n`, where [n] denotes the greatest integer less than or equal to n. Then `sum_(n = 1)^56f(n)` is equal to ______.
Let A = R – {2} and B = R – {1}. If f: A `→` B is a function defined by f(x) = `(x - 1)/(x - 2)` then show that f is a one-one and an onto function.
