Advertisements
Advertisements
प्रश्न
Multiple Choice Question :
Let n(A) = m and n(B) = n then the total number of non-empty relation that can be defined from A to B is ________.
पर्याय
mn
nm
2mn – 1
2mn
उत्तर
Let n(A) = m and n(B) = n then the total number of non-empty relation that can be defined from A to B is 2mn – 1.
APPEARS IN
संबंधित प्रश्न
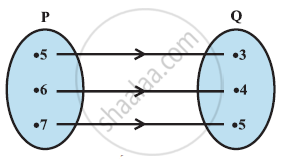
The given figure shows a relationship between the sets P and Q. Write this relation
- in set-builder form.
- in roster form.
What is its domain and range?
Determine the domain and range of the relation R defined by R = {(x, x + 5): x ∈ {0, 1, 2, 3, 4, 5}}.
Determine the domain and range of the relation R defined by
(i) R = [(x, x + 5): x ∈ (0, 1, 2, 3, 4, 5)]
Let R be a relation from N to N defined by R = {(a, b) : a, b ∈ N and a = b2}. Is the statement true?
(a, b) ∈ R implies (b, a) ∈ R
Justify your answer in case.
Let A and B be two sets such that n(A) = 3 and n(B) = 2. If (x, 1), (y, 2), (z, 1) are in A × B, write A and B
If R is a relation on a finite set having n elements, then the number of relations on A is
Write the relation in the Roster Form. State its domain and range
R4 = {(x, y)/y > x + 1, x = 1, 2 and y = 2, 4, 6}
Write the relation in the Roster Form. State its domain and range
R6 = {(a, b)/a ∈ N, a < 6 and b = 4}
Represent the given relation by
(a) an arrow diagram
(b) a graph and
(c) a set in roster form, wherever possible
{(x, y) | x = 2y, x ∈ {2, 3, 4, 5}, y ∈ {1, 2, 3, 4}
Let A = {a, b, c} and R = {(a, a), (b, b), (a, c)}. Write down the minimum number of ordered pairs to be included to R to make it transitive
