Advertisements
Advertisements
प्रश्न
Obtain the differential equation of linear simple harmonic motion.
Derive the differential equation of linear S.H.M.
उत्तर १
Let a particle of mass 'm’ undergo S.H.M. about its mean position O. At any instant ‘t’, displacement of the particle will be ‘x’ as shown in the following figure.
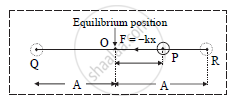
By definition, F = −kx ...(1)
where k is force constant
The acceleration of the particle is given by,
`a=(dv)/(dt)=(d(dx/dt))/dt=(d^2x)/dt^2`
According to Newton’s second law of motion,
F = ma
`therefore F=m((d^2x)/dt^2)` ...(2)
From equations (1) and (2),
`m((d^2x)/dt^2)=-kx`
`therefore (d^2x)/dt^2=-k/mx`
`therefore (d^2x)/dt^2+k/mx=0` ...(3)
where, `k/m=omega^2="constant"`
`therefore (d^2x)/dt^2+omega^2x=0` ...(4)
Equations (3) and (4) represent differential equations of linear S.H.M.
उत्तर २
(i) In a linear S.H.M., the force is directed towards the mean position, and its magnitude is directly proportional to the displacement of the body from the mean position.
∴ f ∝ −x
∴ f = −kx ...(1)
where k is the force constant and x is the displacement from the mean position.
(ii) According to Newton’s second law of motion,
f = ma ….(2)
From equations (1) and (2),
ma = –kx ….(3)
(iii) The velocity of the particle is given by `"v" = "dx"/"dt"`
∴ Acceleration, a = `"dv"/"dt" = ("d"^2"x")/"dt"^2` ...(4)
Substituting equation (4) in equation (3),
`"m" ("d"^2"x")/"dt"^2 = -"kx"`
∴ `("d"^2"x")/"dt"^2 = "k"/"m""x" = 0`
(iv) Substituting `"k"/"m" = omega^2`, where ω is the angular frequency,
∴ `("d"^2"x")/"dt"^2 + omega^2"x" = 0`
This is the differential equation of linear S.H.M.
APPEARS IN
संबंधित प्रश्न
A particle performing linear S.H.M. has a period of 6.28 seconds and a pathlength of 20 cm. What is the velocity when its displacement is 6 cm from mean position?
Define linear S.H.M.
The maximum velocity of a particle performing linear S.H.M. is 0.16 m/s. If its maximum acceleration is 0.64 m/s2, calculate its period.
Two particles perform linear simple harmonic motion along the same path of length 2A and period T as shown in the graph below. The phase difference between them is ___________.
A particle executing linear S.H.M. has velocities v1 and v2 at distances x1 and x2 respectively from the mean position. The angular velocity of the particle is _______
A particle performing linear S.H.M. has the maximum velocity of 25 cm/s and maximum acceleration of 100 cm/ m2. Find the amplitude and period of oscillation. (π = 3.142)
From differential equation of linear S.H.M., obtain an expression for acceleration, velocity and displacement of a particle performing S.H.M.
In SI units, the differential equation of an S.H.M. is `("d"^2"x")/("dt"^2)` = − 36x. Find its frequency and period.
A body of mass m performs linear S.H.M. given by equation, x = P sin cot + Q sin`(omega"t" + pi/2)`. The total energy of the particle at any instant is ______.
A particle performing Linear S.H.M. has a maximum velocity 25 cm/sand maximum acceleration 100 cm/s2. Find the period of oscillations.
What is the rotational analogue of Newton's second law of motion?
The speeds of a particle performing linear SHM are 8 units and 6 units at respective displacements of 6 cm and 8 cm. Find its period and amplitude.
A spring balance has a scale that reads from 0 to 50 kg. The length of the scale is 20 cm. A body suspended from this balance, when displaced and released osillates with a period of 0.6 sec. What is the weight of the body?
