Advertisements
Advertisements
प्रश्न
Obtain the expression for energy stored in the parallel plate capacitor.
उत्तर
- Capacitor not only stores the charge but also stores energy.
- When a battery is connected to the capacitor, electrons of total charge -Q are transferred from one plate to the other plate.
- To transfer the charge, work is done by the battery. This work done is stored as electrostatic potential energy in the capacitor.
- To transfer an infinitesimal charge dQ for a potential difference V, the work done is given by
dW = V dQ ………….(1)
where V = `"Q"/"C"`
The total work done to charge a capacitor is
W = `int_0^"Q" "Q"/"C" "dQ" = "Q"^2/"2C"` .....(2)
This work done is stored as electrostatic potential energy (UB) in the capacitor.
`"U"_"E" = "Q"^2/"2C" = 1/2 "CV"^2` ....(3)
where Q = CV is used. - This stored energy is thus directly proportional to the capacitance of the capacitor and the square of the voltage between the plates of the capacitor.
`"U"_"E" = 1/2 ((ε_0"A")/"d")("Ed")^2 = 1/2 ε_0 ("Ad")"E"^2` ....(4)
where Ad = volume of the space between the capacitor plates. The energy stored per unit volume of space is defined `"U"_"E" = 1/2ε_0 "E"^2` .....(5) - Energy is stored in the electric field existing between the plates of the capacitor. 0nce the capacitor is allowed to discharge, the energy is retrieved.
- The energy density depends only on the electric field and not on the size of the plates of the capacitor.
APPEARS IN
संबंधित प्रश्न
When an AC source is connected to an ideal capacitor, show that the average power supplied by the source over a complete cycle is zero
A capacitor of capacitance C is charged fully by connecting it to a battery of emf E. It is then disconnected from the battery. If the separation between the plates of the capacitor is now doubled, how will the following change?
(i) charge stored by the capacitor.
(ii) Field strength between the plates.
(iii) Energy stored by the capacitor.
Justify your answer in each case.
A capacitor has capacitance C. Is this information sufficient to know what maximum charge the capacitor can contain? If yes, what is this charges? If no, what other information is needed?
A capacitor of capacitance C is charged to a potential V. The flux of the electric field through a closed surface enclosing the capacitor is
A capacitor of capacitance 2⋅0 µF is charged to a potential difference of 12 V. It is then connected to an uncharged capacitor of capacitance 4⋅0 µF as shown in figure . Find (a) the charge on each of the two capacitors after the connection, (b) the electrostatic energy stored in each of the two capacitors and (c) the heat produced during the charge transfer from one capacitor to the other.

A parallel-plate capacitor of capacitance 5 µF is connected to a battery of emf 6 V. The separation between the plates is 2 mm. (a) Find the charge on the positive plate. (b) Find the electric field between the plates. (c) A dielectric slab of thickness 1 mm and dielectric constant 5 is inserted into the gap to occupy the lower half of it. Find the capacitance of the new combination. (d) How much charge has flown through the battery after the slab is inserted?
A capacitor is formed by two square metal-plates of edge a, separated by a distance d. Dielectrics of dielectric constant K1 and K2 are filled in the gap as shown in figure . Find the capacitance.

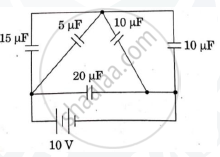
The figure show a network of five capacitors connected to a 10V battery. Calculate the charge acquired by the 5μF capacitor.
Dielectric constant for a metal is ______.
A leaky parallel plate capacitor is filled completely with a material having dielectric constant K = 5 and electric conductivity σ = 7.4 × 10-12 Ω-1 m-1. If the charge on the plate at the instant t = 0 is q = 8.85 µC, then the leakage current at the instant t = 12 s is ______ × 10-1 µA.
