Advertisements
Advertisements
प्रश्न
The force with which the earth attracts an object is called the weight of the object. Calculate the weight of the moon from the following data : The universal constant of gravitation G = 6.67 × 11−11 N−m2/kg2, mass of the moon = 7.36 × 1022 kg, mass of the earth = 6 × 1024 kg and the distance between the earth and the moon = 3.8 × 105 km.
Advertisements
उत्तर
The force between the Earth and the Moon is given by \[F = \frac{GMm}{r^2}\]
Here, M is the mass of the earth; m is the mass of the moon and r is the distance between Earth and Moon.
On substituting the values, we get :
\[F = \frac{6 . 67 \times {10}^{- 11} \times 7 . 36 \times {10}^{22} \times 6 \times {10}^{24}}{3 . 8 \times 3 . 8 \times {10}^{16}}\]
\[= \frac{6 . 67 \times 7 . 36 \times {10}^{35}}{(3 . 8 )^2 \times {10}^{16}}\]
\[ = 20 . 3 \times {10}^{19} = 2 . 03 \times {10}^{20} \]
\[ \approx 2 . 0 \times {10}^{20} N\]
∴ The weight of the moon is \[2 . 0 \times {10}^{20} N\]
APPEARS IN
संबंधित प्रश्न
A body constrained to move along the z-axis of a coordinate system is subject to a constant force F given by
`F = -hati+2hatj+3hatkN`
Where `hati,hatj,hatk` are unit vectors along the x-, y- and z-axis of the system respectively. What is the work done by this force in moving the body a distance of 4 m along the z-axis ?
Is it true that the reaction of a gravitational force is always gravitational, of an electromagnetic force is always electromagnetic and so on?
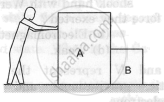
List all the forces acting on the block B in figure.
List all the forces acting on (a) the pulley A, (b) the boy and (c) the block C in figure.
Mark the correct statements :
(a) The nuclear force between two protons is always greater than the electromagnetic force between them.
(b) The electromagnetic force between two protons is always greater than the gravitational force between them.
(c) The gravitational force between two protons may be greater than the nuclear force between them.
(d) Electromagnetic force between two protons may be greater than the nuclear force acting between them.
If all matters were made of electrically neutral particles such as neutrons,
(a) there would be no force of friction
(b) there would be no tension in the string
(c) it would not be possible to sit on a chair
(d) the earth could not move around the sun.
Two charged particles placed at a separation of 20 cm exert 20 N of Coulomb force on each other. What will be the force of the separation is increased to 25 cm?
Find the ratio of the magnitude of the electric force to the gravitational force acting between two protons.
No work is done by a force on an object if
(a) the force is always perpendicular to its velocity
(b) the force is always perpendicular to its acceleration
(c) the object is stationary but the point of application of the force moves on the object
(d) the object moves in such a way that the point of application of the force remains fixed.
A particle moves from a point \[\overrightarrow{r}_1 = \left( 2 m \right) \overrightarrow{ i } + \left( 3 m \right) \overrightarrow{ j } \] to another point
\[\overrightarrow{r}_2 = \left( 3 m \right) \overrightarrow{ i } + \left( 2 m \right) \overrightarrow{ j } \] acts on it. Find the work done by the force on the particle during the displacement.
A block of mass m is kept over another block of mass M and the system rests on a horizontal surface (In the following figure). A constant horizontal force F acting on the lower block produces an acceleration \[\frac{F}{2 \left( m + M \right)}\] in the system, and the two blocks always move together. (a) Find the coefficient of kinetic friction between the bigger block and the horizontal surface. (b) Find the frictional force acting on the smaller block. (c) Find the work done by the force of friction on the smaller block by the bigger block during a displacement d of the system.
A block of mass 2 kg kept at rest on an inclined plane of inclination 37° is pulled up the plane by applying a constant force of 20 N parallel to the incline. The force acts for one second. Find the work done by the force of gravity in that one second if the work done by the applied force is 40 J.
Water falling from a 50-m high fall is to be used for generating electric energy. If \[1 \cdot 8 \times {10}^5 \text{ kg } \] of water falls per hour and half the gravitational potential energy can be converted into electrical energy, how many 100 W lamps can be lit with the generated energy?
The 200 m free-style women's swimming gold medal at Seoul Olympics in 1988 was won by Heike Friendrich of East Germany when she set a new Olympic record of 1 minute and 57⋅56 seconds. Assume that she covered most of the distance with a uniform speed and had to exert 460 W to maintain her speed. Calculate the average force of resistance offered by the water during the swim.
The work done by an applied variable force, F = x + x3 from x = 0 m to x = 2m, where x is displacement, is:
A body is being raised to a height h from the surface of earth. What is the sign of work done by gravitational force?
A cylinder of area 300 cm2 and length 10 cm made of material of specific gravity 0.8 is floated in water with its axis vertical. It is then pushed downward, so as to be just immersed. The work done by the agent who pushes the cylinder into the water is ______ J.
A body is displaced from (0, 0) to (1 m, 1 m) along the path x = y by a force F = (x2`hat"J"` + y`hat"i"`)N. The work done by this force will be:
If a force varies linearly with displacement, the area under the F-s graph forms:
