Advertisements
Advertisements
प्रश्न
PQ is straight line of 13 units. If P has coordinate (2, 5) and Q has coordinate (x, – 7) find the possible values of x.
उत्तर
Here PQ = 13
PQ2 = 132
∴ (x - 2)2 + (-7 - 5)2 = 169
⇒ (x - 2)2 = 169 - 144
= 25 = 52
or
(x - 2) = ± 5
⇒ x = 7 or -3.
APPEARS IN
संबंधित प्रश्न
Find, if point (-2,-1.5) lie on the line x – 2y + 5 = 0
The line `(3x)/5 - (2y)/3 + 1 = 0` contains the point (m, 2m – 1); calculate the value of m.
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the equation of a line, through the centroid and parallel to AB.
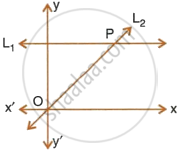
Given equation of line L1 is y = 4.
- Write the slope of line L2 if L2 is the bisector of angle O.
- Write the co-ordinates of point P.
- Find the equation of L2.
P is a point on the line segment AB dividing it in the ratio 2 : 3. If the coordinates of A and Bare (-2,3) and (8,8), find if Plies on the line 7x - 2y =4.
X(4,9), Y(-5,4) and Z(7,-4) are the vertices of a triangle. Find the equation of the altitude of the triangle through X.
Find the equation of a line passing through the intersection of x + 2y + 1= 0 and 2x - 3y = 12 and perpendicular to the line 2x + 3y = 9
Find the equation of a line which is inclined to x axis at an angle of 60° and its y – intercept = 2.
Find the equation of a line that has Y-intercept 3 units and is perpendicular to the line joining (2, – 3) and (4, 2).
Find a general equation of a line which passes through:
(i) (0, -5) and (3, 0) (ii) (2, 3) and (-1, 2).
