Advertisements
Advertisements
प्रश्न
Prove that the points A(–5, 4); B(–1, –2) and C(5, 2) are the vertices of an isosceles right-angled triangle. Find the co-ordinates of D so that ABCD is a square.
उत्तर
We have:
`AB = sqrt((-1 + 5)^2 + (-2 - 4)^2)`
= `sqrt(16 + 36)`
= `sqrt(52)`
`BC = sqrt((-1 + 5)^2 + (-2 - 2)^2)`
= `sqrt(36 + 16)`
= `sqrt(52)`
`AC = sqrt((5 + 5)^2 + (2 - 4)^2)`
= `sqrt(100 + 4)`
= `sqrt(104)`
AB2 + BC2 = 52 + 52 = 104
AC2 = 104
∵ AB = BC and AB2 + BC2 = AC2
∵ ABC is an isosceles right-angled triangle.
Let the coordinates of D be (x, y).
If ABCD is a square, then,
Mid-point of AC = Mid-point of BD
`((-5 + 5)/(2),(4 + 2)/(2)) = ((x - 1)/(2),(y - 2)/(2))`
`0 = (x - 1)/2, 3 = (y - 2)/2`
x = 1, y = 8
Thus, the co-ordinates of point D are (1, 8).
APPEARS IN
संबंधित प्रश्न
Points A and B have co-ordinates (3, 5) and (x, y) respectively. The mid-point of AB is (2, 3). Find the values of x and y.
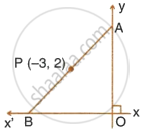
P(–3, 2) is the mid-point of line segment AB as shown in the given figure. Find the co-ordinates of points A and B.
One end of the diameter of a circle is (–2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, –1).
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(2, 6), Q(–4, 1), a : b = 1 : 2
The coordinates of the centroid I of triangle PQR are (2, 5). If Q = (-6, 5) and R = (7, 8). Calculate the coordinates of vertex P.
As shown in the figure. two concentric circles are given and line AB is the tangent to the smaller circle at T. Shown that T is the midpoint of Seg AB

Find the mid-point of the line segment joining the points
(a, b) and (a + 2b, 2a – b)
The points A(−5, 4), B(−1, −2) and C(5, 2) are the vertices of an isosceles right-angled triangle where the right angle is at B. Find the coordinates of D so that ABCD is a square
In what ratio does the point Q(1, 6) divide the line segment joining the points P(2, 7) and R(−2, 3)
Find coordinates of the midpoint of a segment joining point A(–1, 1) and point B(5, –7)
Solution: Suppose A(x1, y1) and B(x2, y2)
x1 = –1, y1 = 1 and x2 = 5, y2 = –7
Using midpoint formula,
∴ Coordinates of midpoint of segment AB
= `((x_1 + x_2)/2, (y_1+ y_2)/2)`
= `(square/2, square/2)`
∴ Coordinates of the midpoint = `(4/2, square/2)`
∴ Coordinates of the midpoint = `(2, square)`
