Advertisements
Advertisements
प्रश्न
As shown in the figure. two concentric circles are given and line AB is the tangent to the smaller circle at T. Shown that T is the midpoint of Seg AB

उत्तर
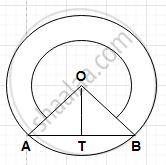
proof OT is perpendicular to AB (as AB = tangent)
other Method -
In ΔAOT, ΔOTB
∠OTB = ∠OTB = 90°
OT = OT = common
OA = OB = radii
∴ ΔAOT ≅ ΔBOT
∴ At = BT ( by cpct)
Now, we know that if a perpendicular is drawn to any chord from the centre, it bisects the chord.
∴ AT = TB
Hence, This midpoint of AB.
APPEARS IN
संबंधित प्रश्न
A(5, 3), B(–1, 1) and C(7, –3) are the vertices of triangle ABC. If L is the mid-point of AB and M is the mid-point of AC, show that : `LM = 1/2 BC`.
(–5, 2), (3, −6) and (7, 4) are the vertices of a triangle. Find the length of its median through the vertex (3, −6).
One end of the diameter of a circle is (–2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, –1).
Points A(–5, x), B(y, 7) and C(1, –3) are collinear (i.e. lie on the same straight line) such that AB = BC. Calculate the values of x and y.
The mid-point of the line segment joining (2a, 4) and (–2, 2b) is (1, 2a + 1). Find the values of a and b.
Prove that the points A(–5, 4); B(–1, –2) and C(5, 2) are the vertices of an isosceles right-angled triangle. Find the co-ordinates of D so that ABCD is a square.
Find the coordinates of point P if P divides the line segment joining the points A(–1, 7) and B(4, –3) in the ratio 2 : 3.
Find the coordinates of midpoint of the segment joining the points (22, 20) and (0, 16).
Find th co-ordinates of the midpoint of the line segment joining P(0, 6) and Q(12, 20).
Find the midpoint of the line segment joining the following pair of point :
(4,7) and (10,15)
Find the midpoint of the line segment joining the following pair of point :
(a+b, b-a) and (a-b, a+b)
Two vertices of a triangle are ( -1, 4) and (5, 2). If the centroid is (0, 3), find the third vertex.
Find the mid-point of the line segment joining the points
(8, −2) and (−8, 0)
The mid-point of the sides of a triangle are (2, 4), (−2, 3) and (5, 2). Find the coordinates of the vertices of the triangle
In what ratio does the point Q(1, 6) divide the line segment joining the points P(2, 7) and R(−2, 3)
The ratio in which the x-axis divides the line segment joining the points A (a1, b1) and B (a2, b2) is
The ratio in which the x-axis divides the line segment joining the points (6, 4) and (1, −7) is
In what ratio does the y-axis divides the line joining the points (−5, 1) and (2, 3) internally
Find coordinates of the midpoint of a segment joining point A(–1, 1) and point B(5, –7)
Solution: Suppose A(x1, y1) and B(x2, y2)
x1 = –1, y1 = 1 and x2 = 5, y2 = –7
Using midpoint formula,
∴ Coordinates of midpoint of segment AB
= `((x_1 + x_2)/2, (y_1+ y_2)/2)`
= `(square/2, square/2)`
∴ Coordinates of the midpoint = `(4/2, square/2)`
∴ Coordinates of the midpoint = `(2, square)`
If A(5, 4), B(–3, –2) and C(1, –8) are the vertices of a ∆ABC. Segment AD is median. Find the length of seg AD:
