Advertisements
Advertisements
प्रश्न
The mid-point of the sides of a triangle are (2, 4), (−2, 3) and (5, 2). Find the coordinates of the vertices of the triangle
उत्तर
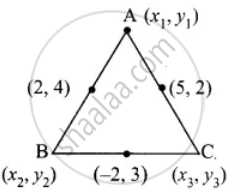
Let the vertices of the ΔABC be A(x1 y1), B(x2, y2) and C(x3, y3)
Mid−point of AB = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
(2, 4) = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
`(x_1 + x_2)/2` = 2
⇒ x1 + x2 = 4 → (1)
`(y_1 + y_2)/2` = 4
⇒ y1 + y2 = 8 → (2)
Mid−point of BC = `((x_2 + x_3)/2, (y_2 + y_3)/2)`
(−2, 3) = `((x_2 + x_3)/2, (y_2 + y_3)/2)`
`(x_2 + x_3)/2` = − 2
⇒ x2 + x3 = − 4 → (3)
`(y_2 + y_3)/2` = 3
⇒ y2 + y3 = 6 → (4)
Mid−point of AC = `((x_1 + x_3)/2, (y_1 + y_3)/2)`
(5, 2) = `((x_1 + x_3)/2, (y_1 + y_3)/2)`
`(x_1 + x_3)/2` = 5
⇒ x1 + x3 = 10 → (5)
`(y_1 + y_3)/2` = 2
⇒ y1 + y3 = 4 → (6)
By adding (1) + (3) + (5) we get
2x1 + 2x2 + 2x3 = 4 – 4 + 10
2(x1 + x2 + x3) = 10
⇒ x1 + x2 + x3 = 5
From (1) x1 + x2 = 4
⇒ 4 + x3 = 5
x3 = 5 – 4 = 1
∴ The vertices of the ΔABC are A(9, 3) B(– 5, 5), C(1, 1)
From (3) x2 + x3 = – 4
⇒ x1 + (– 4) = 5
x1 = 5 + 4 = 9
From (5) ⇒ x1 + x3 = 8
x2 + 10 = 5
x2 = 5 – 10 = – 5
∴ x1 = 9, x2 = – 5, x3 = 1
By adding (2) + (4) + (6) we get
2y1 + 2y2 + 2y3 = 8 + 6 + 4
2(y1 +y2 + y3) = 18
⇒ y1 + y2 + y3 = 9
From (2) ⇒ y1 + y2 = 8
8 + y3 = 9
⇒ y3 = 9 – 8
= 1
From (4)
y2 + y3 = 6
⇒ y1 + 6 = 9
y1 = 9 – 6
= 3
From (6)
y1 + y3 = 4
⇒ y2 + 4
= 9
y2 = 9 – 4
= 5
∴ y1 = 3, y2 = 5, y3 = 1
APPEARS IN
संबंधित प्रश्न
ABCD is a parallelogram where A(x, y), B(5, 8), C(4, 7) and D(2, -4). Find
1) Coordinates of A
2) An equation of diagonal BD
Points A(–5, x), B(y, 7) and C(1, –3) are collinear (i.e. lie on the same straight line) such that AB = BC. Calculate the values of x and y.
Calculate the co-ordinates of the centroid of the triangle ABC, if A = (7, –2), B = (0, 1) and C =(–1, 4).
Point P is the centre of the circle and AB is a diameter . Find the coordinates of point B if coordinates of point A and P are (2, –3) and (–2, 0) respectively.
Find the coordinates of midpoint of the segment joining the points (22, 20) and (0, 16).
Find the midpoint of the line segment joining the following pair of point :
( -3, 5) and (9, -9)
As shown in the figure. two concentric circles are given and line AB is the tangent to the smaller circle at T. Shown that T is the midpoint of Seg AB

Find the mid-point of the line segment joining the points
(−2, 3) and (−6, −5)
Find the co-ordinates of centroid of a triangle if points D(–7, 6), E(8, 5) and F(2, –2) are the mid-points of the sides of that triangle.
Point P is the centre of the circle and AB is a diameter. Find the coordinates of points B if coordinates of point A and P are (2, – 3) and (– 2, 0) respectively.
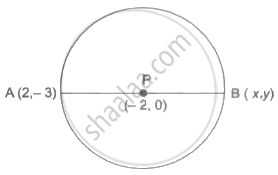
Given: A`square` and P`square`. Let B (x, y)
The centre of the circle is the midpoint of the diameter.
∴ Mid point formula,
`square = (square + x)/square`
⇒ `square = square` + x
⇒ x = `square - square`
⇒ x = – 6
and `square = (square + y)/2`
⇒ `square` + y = 0
⇒ y = 3
Hence coordinates of B is (– 6, 3).
