Advertisements
Advertisements
प्रश्न
Six new employees, two of whom are married to each other, are to be assigned six desks that are lined up in a row. If the assignment of employees to desks is made randomly, what is the probability that the married couple will have nonadjacent desks?
उत्तर
Number of desk occupied by one couple = 1
Only (4 + 1) = 5 persons to be assigned.
∴ Number of ways of assigning these 5 persons = 5! × 2!
Total number of ways of assigning 6 persons = 6!
∴ Probability that a couple has adjacent desk = `(5! xx 2!)/(6!) = 1/3`
So, the probability that the married couple will have no-adjacent desks = `1 - 1/3 = 2/3`.
Hence, the required probability = `2/3`.
APPEARS IN
संबंधित प्रश्न
A coin is tossed twice, what is the probability that at least one tail occurs?
A card is selected from a pack of 52 cards.
- How many points are there in the sample space?
- Calculate the probability that the card is an ace of spades.
- Calculate the probability that the card is
- an ace
- black card.
Three coins are tossed once. Find the probability of getting
- 3 heads
- 2 heads
- at least 2 heads
- at most 2 heads
- no head
- 3 tails
- exactly two tails
- no tail
- atmost two tails.
Check whether the following probabilities P(A) and P(B) are consistently defined
P(A) = 0.5, P(B) = 0.7, P(A ∩ B) = 0.6
Check whether the following probabilities P(A) and P(B) are consistently defined
P(A) = 0.5, P(B) = 0.4, P(A ∪ B) = 0.8
4 cards are drawn from a well-shuffled deck of 52 cards. What is the probability of obtaining 3 diamonds and one spade?
From the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
| S. No. | Name | Sex | Age in years |
| 1. | Harish | M | 30 |
| 2. | Rohan | M | 33 |
| 3. | Sheetal | F | 46 |
| 4. | Alis | F | 28 |
| 5. | Salim | M | 41 |
A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
The number lock of a suitcase has 4 wheels, each labelled with ten digits i.e., from 0 to 9. The lock opens with a sequence of four digits with no repeats. What is the probability of a person getting the right sequence to open the suitcase?
A bag contains 5 red, 6 white and 7 black balls. Two balls are drawn at random. What is the probability that both balls are red or both are black?
If a letter is chosen at random from the English alphabet, find the probability that the letter is a consonant .
Which of the cannot be valid assignment of probability for elementary events or outcomes of sample space S = {w1, w2, w3, w4, w5, w6, w7}:
| Elementary events: | w1 | w2 | w3 | w4 | w5 | w6 | w7 |
| (ii) |
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
\[\frac{1}{7}\]
|
Which of the cannot be valid assignment of probability for elementary events or outcomes of sample space S = {w1, w2, w3, w4, w5, w6, w7}:
| Elementary events: | w1 | w2 | w3 | w4 | w5 | w6 | w7 |
| (iv) |
\[\frac{1}{14}\]
|
\[\frac{2}{14}\]
|
\[\frac{3}{14}\]
|
\[\frac{4}{14}\]
|
\[\frac{5}{14}\]
|
\[\frac{6}{14}\]
|
\[\frac{15}{14}\]
|
Two dice are thrown together. The probability that neither they show equal digits nor the sum of their digits is 9 will be
An urn contains twenty white slips of paper numbered from 1 through 20, ten red slips of paper numbered from 1 through 10, forty yellow slips of paper numbered from 1 through 40, and ten blue slips of paper numbered from 1 through 10. If these 80 slips of paper are thoroughly shuffled so that each slip has the same probability of being drawn. Find the probabilities of drawing a slip of paper that is blue or white
Three-digit numbers are formed using the digits 0, 2, 4, 6, 8. A number is chosen at random out of these numbers. What is the probability that this number has the same digits?
If the letters of the word ALGORITHM are arranged at random in a row what is the probability the letters GOR must remain together as a unit?
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine `P(B ∩ barC)`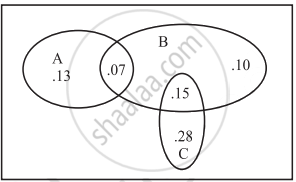
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine P(A ∪ B)
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine `P(A ∩ barB)`
The accompanying Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections (for instance, P(A ∩ B) = .07). Determine P(B ∩ C)
A bag contains 8 red and 5 white balls. Three balls are drawn at random. Find the probability that all the three balls are white
If the letters of the word ASSASSINATION are arranged at random. Find the probability that no two A’s are coming together
In a non-leap year, the probability of having 53 tuesdays or 53 wednesdays is ______.
Without repetition of the numbers, four-digit numbers are formed with the numbers 0, 2, 3, 5. The probability of such a number divisible by 5 is ______.
If the probabilities for A to fail in an examination is 0.2 and that for B is 0.3, then the probability that either A or B fails is ______.
The probability that a person visiting a zoo will see the giraffee is 0.72, the probability that he will see the bears is 0.84 and the probability that he will see both is 0.52.
