Advertisements
Advertisements
प्रश्न
Sketch the graphs of the following functions:
y = `(x^2 + 1)/(x^2 - 4)`
आलेख
उत्तर
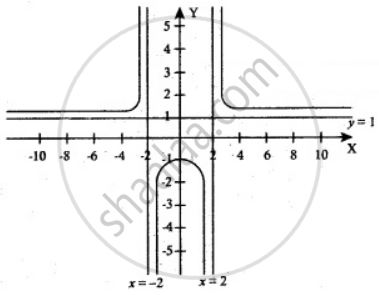
The domain of the given function f(x) is `(-oo, -2) ∪ (-2, 2) ∪ (2, oo)`
ie. x < – 2 or – 2 < x < 2 or x > 2.
Range of f(x) is `(- oo, - 1/4) ∪ (1, oo)`
i.,e. `"f"(x) ≤ - 1/4` or f(x) > 1
Putting y = 0, x is unreal.
Hence, there is no ‘x’ intercept.
By putting x = 0, we get y = `- 1/4`
∴ y intercept is `(0, - 1/4)`
f'(x) = `- (10x)/(x^2 - 4)^2`
f'(x) = 0
⇒ x = 0
∴ The critical point is at x = 0
f'(x) = `(10(x^2 - 4)(3x + 4))/((x^2 - 4)^4`
f'(0) = `- 5/8 < 0`
∴ f(x) is maximum at x = 0.
Hence the local maximum is f(0) = `- 1/4`
No points of inflection exist for the curve.
When x = ± 2, y = `oo`
∴ Vertical asymptotes are x = 2 and x = – 2 and Horizontal asymptote is y = 1.
shaalaa.com
Sketching of Curves
या प्रश्नात किंवा उत्तरात काही त्रुटी आहे का?
पाठ 7: Applications of Differential Calculus - Exercise 7.9 [पृष्ठ ५३]
