Advertisements
Advertisements
प्रश्न
Solve graphically: 3x + 2y ≥ 0
उत्तर
Consider the line whose equation is 3x + 2y = 0. The constant term is zero, therefore this line is passing through the origin.
∴ One point on the line is O = (0, 0).
To find another point, we can give any value of x and get the corresponding value of y.
Put x = 2, we get 6 + 2y = 0 i.e. y = –3
∴ A = (2, –3), is another point on the line. Draw the line OA.
To find the solution set, we cannot check (0,0) as it is already on the line.
We can check any other point which is not on the line.
Let us check the point (1, 1).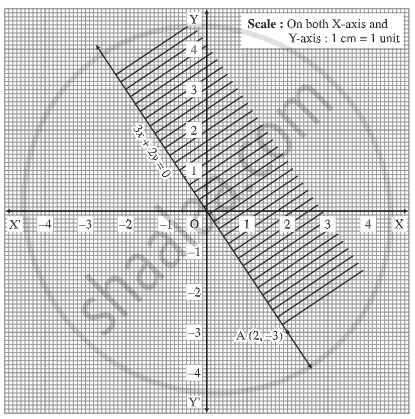
When x = 1, y = 1, then 3x + 2y = 3 + 2 = 5 which is grreater than zer.
∴ 3x + 2y > 0 in this case.
Hence (1, 1) lies in the required region.
Therefore, the required region is the upper side which is shaded in the graph.
This is the solution set of x + 2y > 0.
संबंधित प्रश्न
Solve graphically: x ≥ 0
Solve graphically : x ≤ 0
Solve graphically : x ≥ 0 and y ≥ 0
Solve graphically: x ≤ 0 and y ≥ 0
Solve graphically : x ≤ 0 and y ≤ 0
Solve graphically : x ≥ 0 and y ≤ 0.
Solve graphically: 2x – 3 ≥ 0
Solve graphically : 2y – 5 ≥ 0
Solve graphically : 5x – 3y ≤ 0
Solve graphically : 2x + y ≥ 2 and x – y ≤ 1
Solve graphically : x + y ≥ 6 and x + 2y ≤ 10
Solve graphically : 2x + 3y≤ 6 and x + 4y ≥ 4
The half plane represented by 4x + 3y >14 contains the point
If a corner point of the feasible solutions are (0, 10) (2, 2) (4, 0) (3, 2) then the point of minimum Z = 3x + 2y is
A solution set of the inequality x ≥ 0
Check the ordered points (1, −1), (2, −1) is a solution of 2x + 3y − 6 ≤ 0
If the point (x1, y1) satisfies px - qy < 13, then the solution set represented by the inequation is ______
For the constraint of a linear optimizing function z = 3x1 + 11x2, given by 2x1 + x2 ≤ 2, 4x1 + x2 ≥ 4 and x1, x2 ≥ 0
Solution of the LPP minimize z = 7x + 2y subject to x + y ≥ 60, x - 2y ≥ 0, x + 2y ≤ 120, x, y ≥ 0 is ______
Region represented by the inequalities x ≥ 0, y ≤ 0 is ______.
If S1 and S2 are respectively the sets of local minimum and local maximum points of the function, f(x) = 9x4 + 12x3 - 36x2 + 25, x ∈ R, then ______
Solution set of the inequality y ≥ 0 is ______.
The set of real x satisfying the inequality `(5 - 2x)/3 ≤ x/6 - 5` is [a, ∞). The value of ‘a’ is ______.
Which of the following linear inequalities satisfy the shaded region of the given figure?

The objective function of LPP defined over the convex set attains it optimum value at ______.
