Advertisements
Advertisements
प्रश्न
Solve the system of equations by using the method of cross multiplication:
3x - 2y + 3 = 0,
4x + 3y – 47 = 0
उत्तर
The given equations are:
3x - 2y + 3 = 0 …….(i)
4x + 3y – 47 = 0 …….(ii)
Here` a_1 = 3, b_1 = -2, c_1 = 3, a_2 = 4, b_2 = 3 and c_2 = -47`
By cross multiplication, we have:
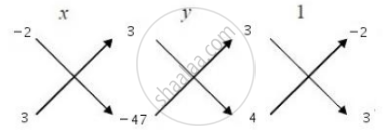
∴ `x/([(−2)×(−47)−3 × 3]) = y/[(3 ×4 −(−47)× 3]) = 1/([3 ×3 −(−2) × 4])`
`⇒x/((94−9)) = y/((12+141) )= 1/((9+8))`
`⇒x/((85)) = y/((153)) = 1/((17))`
`⇒x = 85/17 = 5, y = 153/17 = 9`
Hence, x = 5 and y = 9 is the required solution.
APPEARS IN
संबंधित प्रश्न
Form the pair of linear equations in the following problems and find their solutions (if they exist) by any algebraic method:
A fraction becomes `1/3` when 1 is subtracted from the numerator and it becomes `1/4` when 8 is added to its denominator. Find the fraction.
Solve the following systems of equations:
`x + y/2 = 4`
`x/3 + 2y = 5`
Solve the following systems of equations:
`3x - (y + 7)/11 + 2 = 10`
`2y + (x + 10)/7 = 10`
Solve each of the following systems of equations by the method of cross-multiplication :
x + 2y + 1 = 0
2x − 3y − 12 = 0
Solve each of the following systems of equations by the method of cross-multiplication :
`x(a - b + (ab)/(a - b)) = y(a + b - (ab)/(a + b))`
`x + y = 2a^2`
Solve each of the following systems of equations by the method of cross-multiplication :
`57/(x + y) + 6/(x - y) = 5`
`38/(x + y) + 21/(x - y) = 9`
Solve each of the following systems of equations by the method of cross-multiplication :
`b/a x + a/b y - (a^2 + b^2) = 0`
x + y - 2ab = 0
Solve the system of equations by using the method of cross multiplication:
6x - 5y - 16 = 0,
7x - 13y + 10 = 0
Complete the activity to find the value of x.
3x + 2y = 11 …(i) and 2x + 3y = 4 …(ii)
Solution:
Multiply equation (i) by _______ and equation (ii) by _______.
`square` × (3x + 2y = 11) ∴ 9x + 6y = 33 …(iii)
`square` × (2x + 3y = 4) ∴ 4x + 6y = 8 …(iv)
Subtract (iv) from (iii),
`square` x = 25
∴ x = `square`
Solve 0.4x + 0.3y = 1.7; 0.7 x − 0.2y = 0.8
