Advertisements
Advertisements
प्रश्न
Solve the system of equations by using the method of cross multiplication:
6x - 5y - 16 = 0,
7x - 13y + 10 = 0
उत्तर
The given equations are:
6x - 5y - 16 = 0 …….(i)
7x - 13y + 10 = 0 …….(ii)
Here `a_1 = 6, b_1 = -5, c_1 = -16, a_2 = 7, b_2 = -13 and c_2 = 10`
By cross multiplication, we have:
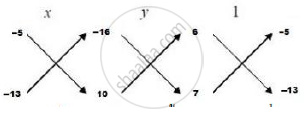
∴ `x/([(−5)×10 −(−16) ×(−13)]) = y/([(−16)×7 −10 × 6]) = 1/([6 ×(−13)−(−5) × 7])`
`⇒x/((−50−208)) = y/((−112−60)) = 1/((−78+35))`
`⇒x/(−258) = y/((−172)) = 1/((43))`
`⇒x = (−258)/(−43) = 6, y = (−172)/(−43) = 4`
Hence, x = 6 and y = 4 is the required solution.
APPEARS IN
संबंधित प्रश्न
For which values of a and b does the following pair of linear equations have an infinite number of solutions?
2x + 3y = 7
(a – b) x + (a + b) y = 3a + b – 2
Solve the following systems of equations:
`x + y/2 = 4`
`x/3 + 2y = 5`
Solve the following systems of equations:
`(7x - 2y)/"xy" = 5`
`(8x + 7y)/"xy" = 15`
Solve each of the following systems of equations by the method of cross-multiplication
3x + 2y + 25 = 0
2x + y + 10 = 0
Solve each of the following systems of equations by the method of cross-multiplication :
`2/x + 3/y = 13`
`5/4 - 4/y = -2`
where `x != 0 and y != 0`
Solve each of the following systems of equations by the method of cross-multiplication
bx + cy = a + b
`ax (1/(a - b) - 1/(a + b)) + cy(1/(b -a) - 1/(b + a)) = (2a)/(a + b)`
Solve each of the following systems of equations by the method of cross-multiplication :
mx – my = m2 + n2
x + y = 2m
Solve the system of equations by using the method of cross multiplication:
`x/6 + y/15 – 4 = 0, x/3 - y/12 – 19/4 = 0`
Solve the system of equations by using the method of cross multiplication:
`a/x - b/y = 0, (ab^2)/x + (a^2b)/y = (a^2 + b^2), where x ≠ 0 and y ≠ 0.`
Solve the following pair of equations:
`x/a + y/b = a + b, x/a^2 + y/b^2 = 2, a, b ≠ 0`
