Advertisements
Advertisements
प्रश्न
State and explain the law used to determine magnetic field at a point due to a current element. Derive the expression for the magnetic field due to a circular current carrying loop of radius r at its centre.
उत्तर
The law which gives the magnetic field at a point due to a current element, is Biot-Savart Law.
It states that,if we consider an infinitesimal element dl of the
conductor,the magnetic field dB due to this
element can be determined at a point P at a distance r from it, as follows:
Let θ be the angle between dl and the displacement vector r.
According to Biot-Savart’s law,the magnitude of the magnetic field dB is proportional tothe current I, the element length |dl|,and inversely proportional to the square of the distance r. Its direction is perpendicular to the plane containing dl and r .
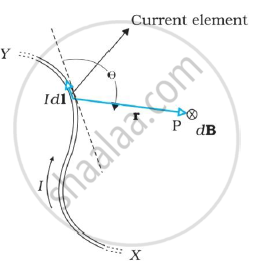
Thus, in vector notation, `d vec( B ) α I (d vec (l)xx vec(r))/(r^3)`
where μ0 / 4π is a constant of proportionality. The above expression holds when the medium is vacuum.The magnitude of this field is,
`|d vec(B) | = (mu_0)/(4pi) (Idlsin theta )/(r^2)`
The proportionality constant in SI units has the exact value,
`(mu_0)/(4pi) = 10^(-7) T - m/A`
We call μ0 the permeability of free space (or vacuum).
Magnetic field at the centre of a circular loop of radius r carrying current I:
We consider a small element of length dl. The `vec (r)` joining the centre of the circle to this element is perpendicular to`d vec(l)` . Hence the magnitude of magnetic field at the centre due to this element (by Biot-Savart Law) is
`dB = (mu_0)/(4 pi) (Idl sin theta)/(r^2) or dB= (mu_0)/(4 pi) (Idl sin 90^circ)/(r^2) = (mu_0)/(4pi) (Idl)/(r^2)`

This field is perpendicular to the plane of the coil (as it is directed along `dvec(l) xx vec (r))`.
Hence, net field due to the entire circular loop, is
`B = int (mu_0)/(4 pi) (Idl )/(r^2) = (mu_0)/(4 pi) (I)/(r^2) int dl = (mu_0)/(4 pi) xx(I)/(r^2) xx 2 pir`
Or B = `(mu_0I)/(2r)`
APPEARS IN
संबंधित प्रश्न
State Biot-Savart law.
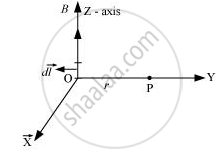
A current I flows in a conductor placed perpendicular to the plane of the paper. Indicate the direction of the magnetic field due to a small element d `vecl` at point P situated at distance `vecr` from the element as shown in the figure.
A long, vertical wire carrying a current of 10 A in the upward direction is placed in a region where a horizontal magnetic field of magnitude 2.0 × 10−3 T exists from south to north. Find the point where the resultant magnetic field is zero.
A long wire with a small current element of length 1 cm is placed at the origin and carries a current of 10 A along the X-axis. Find out the magnitude and direction of the magnetic field due to the element on the Y-axis at a distance 0.5 m from it.
Derive the expression for the magnetic field due to a current carrying coil of radius r at a distance x from the centre along the X-axis.
Two concentric circular loops of radius 1 cm and 20 cm are placed coaxially.
(i) Find mutual inductance of the arrangement.
(ii) If the current passed through the outer loop is changed at a rate of 5 A/ms, find the emf induced in the inner loop. Assume the magnetic field on the inner loop to be uniform.
Biot-Savart law indicates that the moving electrons (velocity v) produce a magnetic field B such that ______.
A circular loop of radius 0.3 cm lies parallel to much bigger circular of radius 20 cm. The centre of the small loop is on the axis of the bigger loop. The distance between their centres is 15 cm. If a current of 2.0 A flows through the smaller loop, then the flux linked with the bigger loop is ______.
Biot-Sawart law indicates that the moving electrons (velocity `overset(->)("v")`) produce a magnetic field B such that
A current carrying loop consists of 3 identical quarter circles of radius R, lying in the positive quadrants of the x-y, y-z and z-x planes with their centres at the origin, joined together. Find the direction and magnitude of B at the origin.
