Advertisements
Advertisements
प्रश्न
The angle between two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 60º. Find the angles of the parallelogram.
Advertisements
उत्तर
In quadrilateral DPBQ:
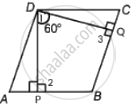
∠1 + ∠2 + ∠B + ∠3 = 360° ...[Angle sum property of quadrilateral]
60° + 90° + ∠B + 90° = 360°
∠B + 240° = 360°
∠B = 360° – 240°
∠B = 120°
Since, ∠ADC = ∠B = 120° ...[Opposite angles of a parallelogram are equal]
∠A + ∠B = 180° ...[Sum of consecutive interior angle is 180°]
∠A + 120° = 180°
∠A = 180° – 120°
∠A = 60°
So, ∠C = ∠A = 60° ...[Opposite angle of a parallelogram are equal]
APPEARS IN
संबंधित प्रश्न
Find x + y + z + w
Find the measure of all the angles of a parallelogram, if one angle is 24° less than twice the smallest angle
If the angles of a quadrilateral are in the ratio 3 : 5 : 9 : 13, then find the measure of the smallest angle.
In the given figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD = 146°, find ∠AOB.
If the diagonals of a rhombus are 18 cm and 24 cm respectively, then its side is equal to
In a rhombus ABCD, if ∠ACB = 40°, then ∠ADB =
In ΔABC, ∠A = 30°, ∠B = 40° and ∠C = 110°. The angles of the triangle formed by joining the mid-points of the sides of this triangle are
Can the angles 110º, 80º, 70º and 95º be the angles of a quadrilateral? Why or why not?
In the following figure, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP = ∠DAP. Prove that AD = 2CD.
The polygon in which sum of all exterior angles is equal to the sum of interior angles is called ______.
