Advertisements
Advertisements
प्रश्न
The area of a rhombus is equal to the area of a triangle. If the base ∆ is 24 cm, its corresponding altitude is 16 cm and one of the diagonals of the rhombus is 19.2 cm. Find its other diagonal.
उत्तर
Area of a rhombus = Area of a triangle Base of triangle = 24 cm and altitude = 16 cm
∴ Area = `1/2 "base" xx "altitude"`
= `1/2 xx 24 xx 16 = 192` cm2
∴ Area of rhombus = 192 cm2
one diagonal = 19.2 cm
second diagonal = `("Area" xx 2)/"one diagonal"`
= `(192 xx 2)/19.2 = (192 xx 10 xx 2)/192 = 20` cm
APPEARS IN
संबंधित प्रश्न
If length of a diagonal of a rhombus is 30 cm and its area is 240 sq cm, find its perimeter.
Find the area of rhombus PQRS shown in the following figure.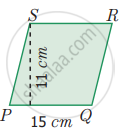
Find the missing value.
| Diagonal (d1) | Diagonal (d2) | Area |
| 19 cm | 16 cm |
The area of the rhombus with side 4 cm and height 3 cm is
The area of the rhombus is 128 sq.cm and the length of one diagonal is 32 cm. The length of the other diagonal is
The figure ABCD is a quadrilateral in which AB = CD and BC = AD. Its area is ______.

Area of a quadrilateral ABCD is 20 cm2 and perpendiculars on BD from opposite vertices are 1 cm and 1.5 cm. The length of BD is ______.
If the diagonals of a rhombus get doubled, then the area of the rhombus becomes ______ its original area.
The walls and ceiling of a room are to be plastered. The length, breadth and height of the room are 4.5 m, 3 m, and 350 cm respectively. Find the cost of plastering at the rate of Rs 8 per m2.
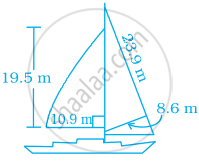
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.