Advertisements
Advertisements
प्रश्न
The bisectors of the equal angles B and C of an isosceles triangle ABC meet at O. Prove that AO bisects angle A.
उत्तर
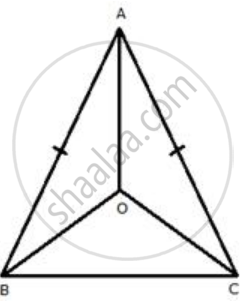
In ΔABC, we have AB = AC
⇒ ∠B = ∠C .......[angles opposite to equal sides are equal]
⇒ `[ 1 ] / [ 2 ]` ∠B = `[ 1 ] / [ 2 ]` ∠C
⇒ ∠OBC = ∠OCB ..........(i)
⇒ OB = OC ...........(ii) [angles opposite to equal sides are equal]
Now,
In ΔABO and ΔACO,
AB = AC ......[Given]
∠OBC = ∠OCB ...[From (i)]
OB = OC ...[From (ii)]
ΔABO ≅ ΔACO ...[ SAS criterion ]
⇒ ∠BAO = ∠CAO .......[ c. p . c .t ]
Therefore, AO bisects ∠BAC.
APPEARS IN
संबंधित प्रश्न
In the figure given below, LM = LN; angle PLN = 110o.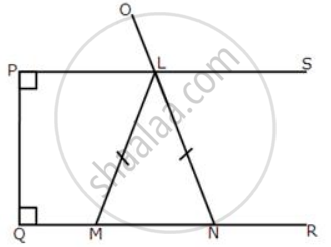
calculate: (i) ∠LMN
(ii) ∠MLN
Calculate x :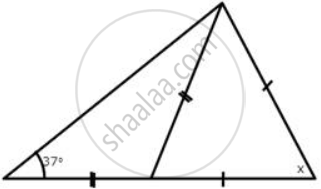
Calculate x :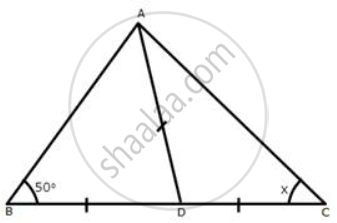
In the given figure; AB = BC and AD = EC.
Prove that: BD = BE.
Prove that a triangle ABC is isosceles, if: bisector of angle BAC is perpendicular to base BC.
In the given figure, AD = AB = AC, BD is parallel to CA and angle ACB = 65°. Find angle DAC.
If the equal sides of an isosceles triangle are produced, prove that the exterior angles so formed are obtuse and equal.
In isosceles triangle ABC, AB = AC. The side BA is produced to D such that BA = AD.
Prove that: ∠BCD = 90°
ABC is a triangle. The bisector of the angle BCA meets AB in X. A point Y lies on CX such that AX = AY.
Prove that: ∠CAY = ∠ABC.
Use the given figure to prove that, AB = AC.
