Advertisements
Advertisements
प्रश्न
The bisects of exterior angle at B and C of ΔABC meet at O. If ∠A = x°, then ∠BOC =
पर्याय
- \[90^\circ + \frac{x^\circ }{2}\]
\[90^\circ - \frac{x^\circ }{2}\]
\[180^\circ + \frac{x^\circ }{2}\]
\[180^\circ - \frac{x^\circ }{2}\]
Advertisements
उत्तर
In the given figure, bisects of exterior angles ∠Band ∠C meet at O and ∠A = x°
We need to find ext. ∠BOC
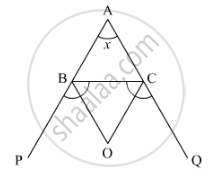
Now, according to the theorem, “if the sides AB and AC of a ΔABC are produced to P and Qrespectively and the bisectors of ∠PBC and ∠QCB intersect at O, therefore, we get,
`∠BOC = 90^\circ - 1/2 ∠A`
Hence, in ΔABC
`∠BOC = 90^\circ - 1/2 ∠A`
`∠BOC = 90^\circ - 1/2 x`
Thus,
`∠BOC = 90^\circ - x/2`
APPEARS IN
संबंधित प्रश्न
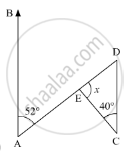
Compute the value of x in the following figure:
Fill in the blank to make the following statement true:
A triangles cannot have more than ......obtuse angles.
Define a triangle.
If one angle of a triangle is equal to the sum of the other two angles, then the triangle is
In a triangle, an exterior angle at a vertex is 95° and its one of the interior opposite angle is 55°, then the measure of the other interior angle is
In ΔPQR, If ∠R > ∠Q then ______.
The correct statement out of the following is 
Match the following:
| Column A | Column B |
| (i) No sides are equal | Isosceles triangle |
| (ii) One right angle | Scalene triangle |
| (iii) One obtuse angle | Right angled triangle |
| (iv) Two sides of equal length | Equilateral triangle |
| (v) All sides are equal | Obtuse angled triangle |
Can we have two acute angles whose sum is an obtuse angle? Why or why not?
Draw a rough sketch of a triangle ABC. Mark a point P in its interior and a point Q in its exterior. Is point A in its exterior or in its interior?
