Question

The figure formed by joining the mid-points of the adjacent sides of a rectangle is a

पर्याय

• square

• rhombus

• trapezium

• none of these

Answer 1

We get a rhombus by joining the mid-points of the sides of a rectangle.

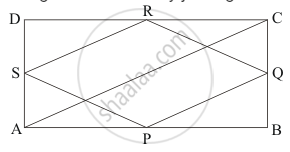
It is given that rectangle ABCD in which P,Q,R and S are the mid-points AB,BC,CD and DA respectively.

PQ,QR,RS and SP are joined.

In ΔABC, P and Q are the mid-points AB and BC respectively.

Therefore,

 PQ || AC and  `PQ = 1/2 AC`……(i)

Similarly, In ΔADC,R and S are the mid-points CD and AD respectively.

Therefore,

 SR || ACand `SR = 1/2 AC `……(ii)

From (i) and (ii), we get

PQ || SR and PQ = SR

Therefore, PQRS is a parallelogram. …… (iii)

Now  ABCD is a rectangle.

Therefore,

AD = BC 

`1/2 AD = 1/2 BC`

AS = BQ …… (iv)

In  ΔAPS and ΔBPQ , we have:

AP = BP (P is the mid point of AB)

∠PAS = ∠PBQ (Each is a right angle)

AS = BQ (From equation (iv))

So, by SAS congruence criteria, we get:

ΔAPS ≅ ΔBPQ

By Corresponding parts of congruent triangles property we have:

PS = PQ …… (v)

From (iii) and (v) we obtain that PQRS is a parallelogram such that PS = PQ.

Thus, the two adjacent sides are equal.

Thus, PQRS is a rhombus .

Hence the correct choice is (b).