Advertisements
Advertisements
प्रश्न
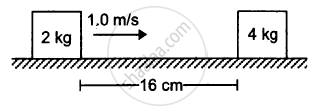
The friction coefficient between the horizontal surface and each of the block shown in figure is 0.20. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest. Take g = 10 m/s2.
उत्तर
Given:
Initial velocity of 2 kg block, v1 = 1.0 m/s
Initial velocity of the 4 kg block, v2 = 0
Let the velocity of 2 kg block, just before the collision be u1.
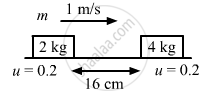
Using the work-energy theorem on the block of 2 kg mass:
The separation between two blocks, s = 16 cm = 0.16 m
\[\therefore \left( \frac{1}{2} \right)m \times u_1^2 - \left( \frac{1}{2} \right)m \times (1 )^2 = - \mu \times mg \times s \]
\[ \Rightarrow u_1 = \sqrt{(1 )^2 - 2 \times 0 . 20 \times 10 \times 0 . 16}\]
\[ \Rightarrow u_1 = 0 . 6 \text{ m/s}\]
As the collision is perfectly elastic, linear momentum is conserved.
Let v1, v2 be the velocities of 2 kg and 4 kg blocks, just after collision.
Using the law of conservation of linear momentum, we can write:
\[m_1 u_1 + m_2 u_2 = m_1 v_1 + m_2 v_2 \]
\[ \Rightarrow 2 \times 0 . 6 + 4 \times 0 = 2 v_1 + 4 v_2 \]
\[ \Rightarrow 2 v_1 + 4 v_2 = 1 . 2 . . . (1)\]
For elastic collision,
Velocity of separation (after collision) = Velocity of approach (before collision)
\[i . e . v_1 - v_2 = + ( u_1 - u_2 )\]
\[ = + (0 . 6 - 0)\]
\[ \Rightarrow v_1 - v_2 = - 0 . 6 . . . (2)\]
\[\text{ Substracting equation (2) from (1), we get: }\]
\[3 v_2 = 1 . 2\]
\[ \Rightarrow v_2 = 0 . 4 \text{ m/s}\]
\[ \therefore v_1 = - 0 . 6 + 0 . 4 = - 0 . 2 \text{ m/s} \]
Let the 2 kg block covers a distance of S1.
∴ Applying work-energy theorem for this block, when it comes to rest:
\[\left( \frac{1}{2} \right) \times 2 \times (0 )^2 + \left( \frac{1}{2} \right) \times 2 \times (0 . 2 )^2 = - 2 \times 0 . 2 \times 10 \times S_1 \]
\[ \Rightarrow S_1 = 1 cm .\]
Let the 4 kg block covers a distance of S2.
Applying work energy principle for this block:
\[\left( \frac{1}{2} \right) \times 4 \times (0 )^2 - \left( \frac{1}{2} \right) \times 4 \times (0 . 4 )^2 = - 4 \times 0 . 2 \times 10 \times S_2 \]
\[ \Rightarrow 2 \times 0 . 4 \times 0 . 4 = 4 \times 0 . 2 \times 10 \times S_2 \]
\[ \Rightarrow S_2 = 4 \text{ cm}\]
Therefore, the distance between the 2 kg and 4 kg block is given as,
S1 + S2 = 1 + 4 = 5 cm
APPEARS IN
संबंधित प्रश्न
Two bodies make an elastic head-on collision on a smooth horizontal table kept in a car. Do you expect a change in the result if the car is accelerated in a horizontal road because of the non inertial character of the frame? Does the equation "Velocity of separation = Velocity of approach" remain valid in an accelerating car? Does the equation "final momentum = initial momentum" remain valid in the accelerating car?
Suppose we define a quantity 'Linear momentum' as linear momentum = mass × speed.
The linear momentum of a system of particles is the sum of linear momenta of the individual particles. Can we state principle of conservation of linear momentum as "linear momentum of a system remains constant if no external force acts on it"?
Consider the following two statements:
(A) Linear momentum of a system of particles is zero.
(B) Kinetic energy of a system of particles is zero.
A bullet hits a block kept at rest on a smooth horizontal surface and gets embedded into it. Which of the following does not change?
In an elastic collision
(a) the kinetic energy remains constant
(b) the linear momentum remains constant
(c) the final kinetic energy is equal to the initial kinetic energy
(d) the final linear momentum is equal to the initial linear momentum.
A ball hits a floor and rebounds after an inelastic collision. In this case
(a) the momentum of the ball just after the collision is same as that just before the collision
(b) the mechanical energy of the ball remains the same during the collision
(c) the total momentum of the ball and the earth is conserved
(d) the total energy of the ball and the earth remains the same
A uranium-238 nucleus, initially at rest, emits an alpha particle with a speed of 1.4 × 107m/s. Calculate the recoil speed of the residual nucleus thorium-234. Assume that the mass of a nucleus is proportional to the mass number.
A man of mass 50 kg starts moving on the earth and acquires a speed 1.8 m/s. With what speed does the earth recoil? Mass of earth = 6 × 1024 kg.
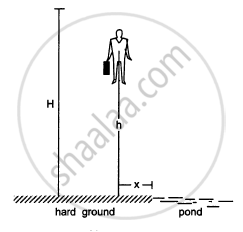
A man of mass M having a bag of mass m slips from the roof of a tall building of height H and starts falling vertically in the following figure. When at a height h from the ground, the notices that the ground below him is pretty hard, but there is a pond at a horizontal distance x from the line of fall. In order to save himself he throws the bag horizontally (with respect to himself) in the direction opposite to the pond. Calculate the minimum horizontal velocity imparted to the bag so that the man lands in the water. If the man just succeeds to avoid the hard ground, where will the bag land?
A ball of mass 50 g moving at a speed of 2.0 m/s strikes a plane surface at an angle of incidence 45°. The ball is reflected by the plane at equal angle of reflection with the same speed. Calculate (a) the magnitude of the change in momentum of the ball (b) the change in the magnitude of the momentum of the ball.
In a typical Indian Bugghi (a luxury cart drawn by horses), a wooden plate is fixed on the rear on which one person can sit. A bugghi of mass 200 kg is moving at a speed of 10 km/h. As it overtakes a school boy walking at a speed of 4 km/h, the boy sits on the wooden plate. If the mass of the boy is 25 kg, what will be the plate. If the mass of the boy is 25 kg, what will be the new velocity of the bugghi ?
A ball of mass 0.50 kg moving at a speed of 5.0 m/s collides with another ball of mass 1.0 kg. After the collision the balls stick together and remain motionless. What was the velocity of the 1.0 kg block before the collision?
A 60 kg man skating with a speed of 10 m/s collides with a 40 kg skater at rest and they cling to each other. Find the loss of kinetic energy during the collision.
A bullet of mass 20 g travelling horizontally with a speed of 500 m/s passes through a wooden block of mass 10.0 kg initially at rest on a level surface. The bullet emerges with a speed of 100 m/s and the block slides 20 cm on the surface before coming to rest. Find the friction coefficient between the block and the surface (See figure).
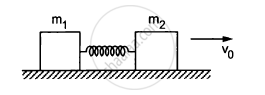
Two blocks of masses m1 and m2 are connected by a spring of spring constant k (See figure). The block of mass m2 is given a sharp impulse so that it acquires a velocity v0 towards right. Find (a) the velocity of the centre of mass, (b) the maximum elongation that the spring will suffer.
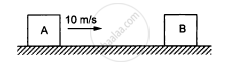
The blocks shown in figure have equal masses. The surface of A is smooth but that of Bhas a friction coefficient of 0.10 with the floor. Block A is moving at a speed of 10 m/s towards B which is kept at rest. Find the distance travelled by B if (a) the collision is perfectly elastic and (b) the collision is perfectly inelastic.
A sphere starts rolling down an incline of inclination θ. Find the speed of its centre when it has covered a distance l.
A solid sphere of mass m is released from rest from the rim of a hemispherical cup so that it rolls along the surface. If the rim of the hemisphere is kept horizontal, find the normal force exerted by the cup on the ball when the ball reaches the bottom of the cup.
