Advertisements
Advertisements
प्रश्न
The length of the diagonals of a rhombus is in ratio 4 : 3. If its area is 384 cm2, find its side.
Advertisements
उत्तर
Let the lengths of the diagonals of a rhombus are 4x, 3x.
∴ Area of the rhombus = `1/2 xx ("Product of its diagonals")`
= `1/2 (4x xx 3x) = 384` (given)
⇒ `6x^2 = 384 ⇒ x^2 = 64`
⇒ x = 8 cm
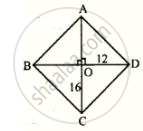
∴ Diagonals are `4 xx 8 = 32` cm and `3(8) = 24` cm.
∴ OC = 16 cm and OD = 12 cm
∴ Side DC = `sqrt("OC"^2 + "OD"^2)`
∴ Side DC = `sqrt(16^2 + 12^2)` [By Pythagoras Theorem in ΔDOC]
= `sqrt(256 + 144) = sqrt(400) = 20` cm
Hence , side of the rhombus = 20 cm.
APPEARS IN
संबंधित प्रश्न
Lengths of the diagonals of a rhombus are 15 cm and 24 cm, find its area.
The area of a rhombus is equal to the area of a triangle. If the base ∆ is 24 cm, its corresponding altitude is 16 cm and one of the diagonals of the rhombus is 19.2 cm. Find its other diagonal.
Find the missing value.
| Diagonal (d1) | Diagonal (d2) | Area |
| 26 m | 468 sq.m |
The area of a rhombus is 100 sq.cm and length of one of its diagonals is 8 cm. Find the length of the other diagonal
The area of the rhombus is 128 sq.cm and the length of one diagonal is 32 cm. The length of the other diagonal is
A man has to build a rhombus shaped swimming pool. One of the diagonal is 13 m and the other is twice the first one. Then find the area of the swimming pool and also find the cost of cementing the floor at the rate of ₹ 15 per sq.cm
What is the area of the rhombus ABCD below if AC = 6 cm and BE = 4 cm?
Area of a rhombus = `1/2` product of ______.
The area of a rectangular field is 48 m2 and one of its sides is 6 m. How long will a lady take to cross the field diagonally at the rate of 20 m/minute?
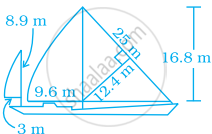
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.
