Advertisements
Advertisements
प्रश्न
The longest side of a triangle is three times the shortest side and third side is 2 cm shorter than the longest side if the perimeter of the triangles at least 61 cm, find the minimum length of the shortest-side.
उत्तर
Let the shortest side of the triangle be x cm.
Then, the longest side will be 3x and the third side will be 3x − 2.
\[\therefore \text{ Perimeter of the triangle } \geq 61\]
\[ \Rightarrow x + 3x + 3x - 2 \geq 61\]
\[ \Rightarrow 7x \geq 61 + 2\]
\[ \Rightarrow x \geq 9 (\text{ Dividing throughout by } 7)\]
\[\text{ Hence, the minumum length of the shortest side is 9 cm } .\]
APPEARS IN
संबंधित प्रश्न
Solve the following system of inequalities graphically: 2x + y≥ 6, 3x + 4y ≤ 12
Solve the following system of inequalities graphically: x + y≥ 4, 2x – y > 0
Solve the following system of inequalities graphically: x + y ≤ 6, x + y ≥ 4
Solve the following system of inequalities graphically: 2x + y≥ 8, x + 2y ≥ 10
Solve the following system of inequalities graphically: 5x + 4y ≤ 20, x ≥ 1, y ≥ 2
Solve the following system of inequalities graphically: 3x + 4y ≤ 60, x + 3y ≤ 30, x ≥ 0, y ≥ 0
Solve the following system of inequalities graphically: 2x + y≥ 4, x + y ≤ 3, 2x – 3y ≤ 6
Solve the following system of inequalities graphically: x – 2y ≤ 3, 3x + 4y ≥ 12, x ≥ 0, y ≥ 1
Solve the following system of inequalities graphically: 4x + 3y ≤ 60, y ≥ 2x, x ≥ 3, x, y ≥ 0
Solve the following system of inequalities graphically: 3x + 2y ≤ 150, x + 4y ≤ 80, x ≤ 15, y ≥ 0, x ≥ 0
Find all pairs of consecutive odd natural number, both of which are larger than 10, such that their sum is less than 40.
The marks scored by Rohit in two tests were 65 and 70. Find the minimum marks he should score in the third test to have an average of at least 65 marks.
A solution is to be kept between 86° and 95°F. What is the range of temperature in degree Celsius, if the Celsius (C)/ Fahrenheit (F) conversion formula is given by\[F = \frac{9}{5}C + 32\]
A solution is to be kept between 30°C and 35°C. What is the range of temperature in degree Fahrenheit?
A company manufactures cassettes and its cost and revenue functions for a week are \[C = 300 + \frac{3}{2}x \text{ and } R = 2x\] respectively, where x is the number of cassettes produced and sold in a week. How many cassettes must be sold for the company to realize a profit?
A solution of 8% boric acid is to be diluted by adding a 2% boric acid solution to it. The resulting mixture is to be more than 4% but less than 6% boric acid. If there are 640 litres of the 8% solution, how many litres of 2% solution will have to be added?
Write the solution set of the equation |2 − x| = x − 2.
Write the set of values of x satisfying |x − 1| ≤ 3 and |x − 1| ≥ 1.
Write the solution set of the inequation \[\left| \frac{1}{x} - 2 \right| > 4\]
Write the number of integral solutions of \[\frac{x + 2}{x^2 + 1} > \frac{1}{2}\]
Write the solution of set of\[\left| x + \frac{1}{x} \right| > 2\]
Write the solution set of the inequation |x − 1| ≥ |x − 3|.
Solve each of the following system of equations in R.
\[5x - 7 < 3\left( x + 3 \right), 1 - \frac{3x}{2} \geq x - 4\]
Find the graphical solution of the following system of linear inequations:
2x + 3y ≥ 12, – x + y ≤ 3, x ≤ 4, y ≥ 3
Find the graphical solution of the following system of linear inequations:
3x + 2y ≤ 1800, 2x + 7y ≤ 1400
Solve the following system of inequalities graphically.
2x – y ≥ 1, x – 2y ≤ – 1
Find the linear inequalities for which the shaded region in the given figure is the solution set.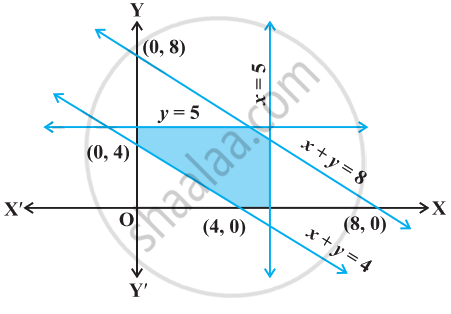
Show that the following system of linear inequalities has no solution x + 2y ≤ 3, 3x + 4y ≥ 12, x ≥ 0, y ≥ 1
Solution set of x ≥ 0 and y ≤ 0 is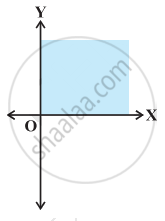
Solution set of x ≥ 0 and y ≤ 1 is