Question

The radius of the base of a right circular cone of semi-vertical angle α is r. Show that its volume is \[\frac{1}{3} \pi r^3\] cot α and curved surface area is πr² cosec α.

 

Answer 1

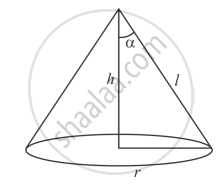
\[\sin \alpha = \frac{r}{l}\]

\[ \Rightarrow r \cos ec \ \alpha = l\]

\[\tan \alpha = \frac{r}{h}\]

\[ \Rightarrow\text {  r cot } \alpha = h\]

`"volume = 1/3 pir^2h"`

`=1/3 pir^2 . r cost \ alpha`

`=1/3 pir^2 cot \ alpha`

Surface area = `pirl`      

                     = πr² cosec α.                   

                    = πr² cosec α.