Advertisements
Advertisements
प्रश्न
Two cubes each of volume 125 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
उत्तर
Let the edge of the cube be a.
As,
Volume of the cube = 125 cm3
⇒ a3 = 125
`=> a = root(3)(125)`
`=> a = 5 "cm"`
So,
Length of the resulting cuboid, `l = 2xx5 = 10 "cm",`
Breath of the resulting cuboid, b = 5 cm and
Height of the resulting cuboid, h = 5 cm
Now,
Surface area of the resulting cuboid = 2 (lb + bh + hl)
`= 2 xx (10xx5+5xx5+5xx10)`
`= 2xx(50+25+50)`
`=2xx125`
`=250 cm2`
APPEARS IN
संबंधित प्रश्न
Metal spheres each of radius 2cm are packed into a rectangular box of internal dimension 16cm x 8cm x 8cm when 16 spheres are packed the box is filled with preservative liquid. Find volume of this liquid?
A copper sphere of diameter 18 cm is drawn into a wire of diameter 4 mm. Find the length of the wire.
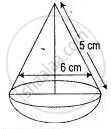
The given figure represents a hemisphere surmounted by a conical block of wood. The diameter of their bases is 6 cm each and the slant height of the cone is 5 cm. Calculate:
(i) the height of the cone.
(ii) the vol. of the solid.
If the radius of base of a right circular cylinder is halved, keeping the height same, the ratio of the volume of the reduced cylinder to that of the original cylinder is ______.
Given that 1 cu. cm of marble weighs 25 g, the weight of a marble block of 28 cm in width and 5 cm thick, is 112 kg. The length of the block is ______.
A solid piece of iron in the form of a cuboid of dimensions 49 cm × 33 cm × 24 cm, is moulded to form a solid sphere. The radius of the sphere is ______.
A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of two parts.
Two solid cones A and B are placed in a cylinderical tube as shown in the figure. The ratio of their capacities are 2:1. Find the heights and capacities of cones. Also, find the volume of the remaining portion of the cylinder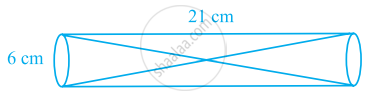
A hemispherical bowl of internal radius 9 cm is full of liquid. The liquid is to be filled into cylindrical shaped bottles each of radius 1.5 cm and height 4 cm. How many bottles are needed to empty the bowl?
A solid right circular cone of height 120 cm and radius 60 cm is placed in a right circular cylinder full of water of height 180 cm such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is equal to the radius of the cone.
