Question

Use Huygens’ geometrical construction to show the propagation of plane wavefront a rarer medium (1) to a denser medium (2) undergoing refraction.

Hence derive Snell’s law of refraction.

Answer 1

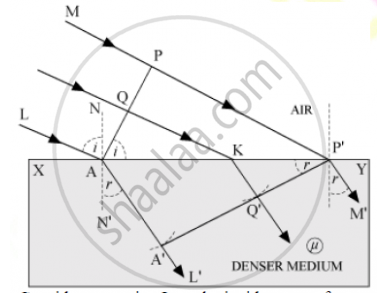
Consider any point Q on the incident wavefront.

Suppose when disturbance from point P on incident wavefront reaches point P’ on the refracted wavefront, the disturbance from point Q reaches Q’on the refracting surface XY. Since represents the refracted wavefront, the time taken by light to travel from a point on incident wavefront to the corresponding point on refracted wavefront should always be the same. Now, time taken by light to go from Q to Q’ will be

`t =(QK)/c +(KQ')/v   ......... (1)`

In right-angled ΔAQK, ∠QAK = i

∴ QK = AK sin i … (ii)

In right-angled Δ P′Q′K,<q′p′k p=""></q′p′k>

KQ′ = KP′ sin r ………….(iii)

Substituting (ii) and (iii) in equation (i),

`t= (AK sin i)/c +(KP'sinr)/v`

`t =(AK sini)/c +((AP' -AK)sinr)/v`

`or, t =(Ap')/v sinr +(sini/c - sinr/v) AK  ...... (4)`

The rays from different points on the incident wavefront will take the same time to reach the corresponding points on the refracted wavefront i.e., given by equation (iv) is independent of AK. It will happen so, if

`sini/c -sinr/v =0`

`sini/sinr  = c/v`

However, `c/v = n`

`sini/sinr = n`

This is the Snell’s law for refraction of light.